Suppose one has a binary asymmetric channel K : S → R representedbyand1Prs(r|1) = 2 (r = 1) + (r = 0)212Pas (10) = (r = 1) + (r = 0)33(a) Represent PRIS(rs) in a table.(b) Use the assumption, that1Ps(0) and Ps(1)3==12|31/32/31/21/2to compute PS,R(s,r).(c) What are PR(r), and PSR(s|r) for all r, s = {0, 1}?(d) What are the maximum likelihood and maximum a posteriori de-cisions for r = 1?

Hello! As you have posted more than 3 sub parts, we are answering the first 3 sub-parts. In case…

Answered: Suppose one has a binary asymmetric…

Math

Probability

Suppose one has a binary asymmetric channel K: S→ R represented by and Pris(r|1)-(-1)+(-0) = = = 1/3 2/3 1/2

A First Course in Probability (10th Edition)

10th Edition

ISBN:9780134753119

Author:Sheldon Ross

Publisher:Sheldon Ross

Chapter1: Combinatorial Analysis

Section: Chapter Questions

Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...

See similar textbooks

Related questions

Q: 5. In a supermarket, the weights of apples and pears have approximately normal distributions. The…

A: Let X be the weights of apples follows normal distribution with mean 150 and standard deviation 15.…

Q: Let A, B, C be three events in the sample space S. The expression for the event that at least one of…

A: GivenA,B,C,be the three events in the sample space S

Q: A number cube is rolled three times. An outcome is represented by a string of the sort OEE (meaning…

A: Given 8 outcomes isOEE,EEO,OOE,OEO,EOO,OOO,EOE,EEETotal no.of outcomes=8Where,'O'=odd number'E'=Even…

Q: An audio company sells microphones for $110 per unit. Its fixed costs are $152,000 per year, and the…

A: Break even volume explain the minimum number of sales require to make the profit. It is calculated…

Q: 27. If {Xn, n ≥ 1} are independent random variables, show that the radius of convergence of the…

A: Answer :Consider ∑nXnzn where Xn = Xn(ω) is an independent (complex) random variable and z is…

Q: An experiment is conducted for which the sample space is S = (S₁,S2,S3,S4,S5}. Is the following…

A: outcomes s1 s2 s3 s4 s5 probabilities 1/3 1/6 1/8 1/10 1/15 is the probability assignment…

Q: An entry into the UK lottery consists of a list of six numbers. During the draw, six numbered balls…

A: A combination is a selection of items from a group of items, where the order of selection is not…

Q: king, (b) all cards are of one suit, (c) all cards are of -85 Find the probability of at least two…

A: Given::- the probability of at least two 7's in four tosses of a pair of dice.

Q: Four balls are randomly selected, without replacement, from a bag that contains 10 balls numbered…

A: Given::- Four balls are randomly selected, without replacement, from a bag that contains 10 balls…

Q: 6. Choose 2 points (X, Y) uniformly at random from the circle. Find the expected area of the…

Q: A cook is preparing fruit jam for the festival next week. She has bought 40 apples from the farmer's…

A: To solve the problem we will use Hypergeometric probability distribution formula which is as follows…

Q: 7.63 Adult males have normally distributed heights with mean equal to 70 in and standard deviation…

A: 7.63 Answer:-Given, mean = 70 in And standard deviation= 3 in The distribution is normally…

Q: Teresa had a gross income of $91,979 in 2010. What was her net income in 2010? Use the income tax…

Q: To get from Lime End to Blackchurch, there are three public transport options: you can travel via…

A: given data event A : bus is running event B : tube is running event c : monorail is running P(A) =…

Q: A box has four cards numbered 1, 2, 3 and 4. Keiko will toss a coin once and record the toss as…

A: From given A box has four cards numbered 1, 2, 3 and 4. And let H=Heads T=Tails

Q: You generate three random numbers from (0, 1). Let X be the smallest of these three numbers and Y…

A: Three random numbers are drawn from a distribution with parameters (0,1). As each value has equal…

Q: Suppose a box contains 18 balls numbered 1 through 6, three balls with each number. When 5 balls are…

A: It is given that a box contains 18 balls numbered 1 through 6, three balls with each number.

Q: Given P(A) = 0.42, P(B) = 0.21, and P(B|A) = 0.27, what is P(A and B)?

A: Given that Given P(A) = 0.42, P(B) = 0.21, and P(B|A) = 0.27, Then P(A and B)

Q: 5. Assume you are shooting at a target centred at the origin (0,0), but bullets land at a random…

A: Hi! Thank you for the question, As per the honor code, we are allowed to answer three sub-parts at a…

Q: A survey of 10 fast-food restaurants noted the number of calories in a mid-sized hamburger. The…

A: Given information- We have given a survey of 10 fast-food restaurants noted the number of calories…

Q: Suppose there are two buses, the Alphabus and the Betabus, running from station P to station Q along…

A: there are two buses : Alphabus and Betabus A: Aplhabus is running B : Betabus is running P(A) =…

Q: 11) According to a recent report, 68.3% of men and 64.1% of women in the US are overweight. Given…

A: Given: 68.3% of men are overweight. 64.1% of women are overweight. 49.3% of Americans are men 50.7%…

Q: Q4 The overall distance traveled by a golf ball is tested by hitting the ball with iron. Eight…

A: Given::- The overall distance traveled by a golf ball is tested by hitting the ball with iron. Eight…

Q: The table below lists the number of head and neck injuries for ice hockey players' exposures wearing…

A: Head and Face (A) Concussions(B) Neck(C) Other(O) Total Half(H) 43 12 22 20 97 Full(F) 37 30…

Q: For the following performance function and values shown for the variables, determine the safety…

A: The mentioned equation is, Z=f(w,x,y)=w3×x2+x×y2-5×w2×yμz=fμw,μx,μy

Q: 240 x The weekly average costs, in dollars, of the We-Are-Drones is given by C +57 + where I 19…

Q: Q.3.@ Jf X'is normally distributed with mean and variance fo.1, then find P(|X-21) 20.0 taken from D…

A: Solution: a) Let X is normally distributed with mean 2 and variance 0.1. X~N(μ=2, σ2=0.1)

Q: percent spend between $10 7.65 The mean grade on a final examination was 72 and the standard…

A: Given::- The mean grade on a final examination was 72 and the standard deviation was 9. The top 10%…

Q: 7. Suppose that the time till a beggar gets something is exponentially distributed with mean 2…

Q: A box contains a very large number of red, white, blue, and yellow marbles in the ratio 4: 3:2:1…

A: Given::- A box contains a very large number of red, white, blue, and yellow marbles in the ratio…

Q: A baseball player has a batting average of 0.36. What is the probability that he has exactly 4 hits…

A: Given that Number of trials n=7 Success of probability p=0.36 X~bin(n,p)

Q: A jar contains two white and three black balls. A sample of size 4 is made.What is the probability…

A: A jar contains two white and three black balls. A sample of size 4 is made.

Q: Consider 92 independent observations. The probability of event A in a particular experiment is 0.03.…

A: From given we have : Event A happens in a particular experiment is 0.03 P(A)=0.03 Therefore, the…

Q: A machine generates rectangular tiles with randomly varying length (L) and width (W). Let the length…

A: Hi! Thank you for the question, As per the honor code, we are allowed to answer three sub-parts at a…

Q: Discrete Mathematics (Counting theory) We want to spread a face-to-face exam among 24 students…

A: Given::- We want to spread a face-to-face exam among 24 students over three days: Wednesday,…

Q: 9 Let X and Y be two continuous random variables with the joint proba- bility density f(x, y) =…

A: 7.9 fx,y=xe-xy+1, x,y>0 Consider, fx=∫yfx,ydy =∫y=0∞xe-xy+1dy =xe-xe-xy-xy=0∞ =xe-x0-1-x…

Q: Suppose we toss a fair coin three times and denote outcomes in the sample space S by listing, in…

A: Given::- Suppose we toss a fair coin three times and denote outcomes in the sample space S by…

Q: An urn contains 7 red, 3 white, and 6 black balls. One ball is drawn from the urn, it is replaced,…

A: It is given that Red balls = 7 White balls = 3 Black balls = 6 Total balls = 16

Q: When I open a bank account I am allocated a 4-digit personal identification number (which may begin…

A: The cardinality of set is a the number of elements in that set. For example, let us consider the a…

Q: Let X be a geometric random variable with parameter p = 0.1 and range space x 1 Evaluate th habilib:…

A: Let X be the geometric random variable with parameter p=0.1 PX=x=1-px-1p, x=1,2,3,...…

Q: The National Highway Traffic Safety Administration (NHTSA) provides ratings on all tire lines…

A: Hi! Thank you for the question, As per the honor code, we are allowed to answer one question at a…

Q: A marble is selected from a bag containing eight marbles numbered 1 to 8. The number on the marble…

A: a) There is total eight marbles, numbered 1 to 8.

Q: deviation? 7.67 If X is the mean and s is the standard deviation of a set of normally distributed…

A: Given::- standard deviation of a set of normally distributed measurements, what percentage of the…

Q: The bowler bowls three balls and is aiming to hit the "leg stump" (the stick on the Right). (Ball…

A: Introduction: We are given a bowling stat of 3 bowlers. The target is the leg stump. We need to…

Q: Find the mean, the median, and the mode(s), if any, for the given data. Round noninteger means to…

A: we have to find out the mean , median and mode for the given data.39,30,6,25,9,21,28,10data arrange…

Q: 6.87 (a) chosen at random (a) none will be defective, (b) 1 will be defective, and (c) at least 2…

A: Given::- Set up a sample space for the outcomes of 2 tosses of a fair coin, using 1 to represent…

Q: The figure below shows graphs of the total cost function and the total revenue functions for a…

A: At first note that Blue line indicates Total Revenue(Y axis) and Red Line indicates total cost…

Q: Suppose each license plate in a certain state has four letters followed by two digits. The letters…

A: given data, each license plate in a certain state has four letters followed by two digitthe…

Q: A bag contains 3 nickels, 5 dimes, and 4 quarters. If you draw 3 coins at random from the bag,…

A: It is given that Nickels coins = 3 Dimes coins = 5 Quarters coins = 4 Total coins = 12

Q: 3E1. An interval estimate is a range of values us to estimate (a) the shape of the population…

A: Here, need to find out the correct choice for the given statements Note: According to Bartleby…

Question

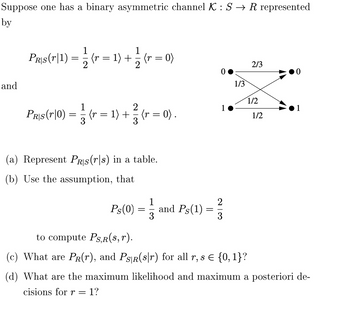
Suppose one has a binary asymmetric channel K : S → R represented
by
and
1
Prs(r|1) = 2 (r = 1) + (r = 0)
2
1
2
Pas (10) = (r = 1) + (r = 0)
3
3
(a) Represent PRIS(rs) in a table.
(b) Use the assumption, that
1
Ps(0) and Ps(1)
3
=
=
1
2|3
1/3
2/3
1/2
1/2
to compute PS,R(s,r).
(c) What are PR(r), and PSR(s|r) for all r, s = {0, 1}?
(d) What are the maximum likelihood and maximum a posteriori de-
cisions for r = 1?

Expert Solution

This question has been solved!

Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.

SEE SOLUTION Check out a sample Q&A here

Step 1

VIEW

Step 2

VIEW

Step 3

VIEW

Step by step

Solved in 3 steps

SEE SOLUTION Check out a sample Q&A here

Follow-up Questions

Read through expert solutions to related follow-up questions below.

Follow-up Question

I very sorry but I think on a you are wrong as the sum of the should be 1. I was expecting the below

Representation of PR|Sr|s in a table form:

r\|s	0	1
0	2/3	1/3
1	1/2	1/2

You don't need to compte, I was able to do it.

Let me know if you are able to give me a answer to question d, of if you still would like me to send a new quetion

Solution

by Bartleby Expert

SEE SOLUTION

Similar questions

Suppose a, = (1 -1 1 1) 5. az = (1 0 1 0) %3D %3D az = (1 1 1 1)''a¸ = (3 2 3 0)" - Please find out whether a1,a2,a3,&4 are linear independent or not?
Determine whether the following sets are linearly dependent or linearlyindependent. (a) {(; )( )} . -9} in Maxa(R) 4 (b) {(- ).(- 9G -)} in M2x2(R) 4 (c) {x 3 + 2x2, -x2 + 3x + 1, x3 – x2 + 2x – 1} in P3(R) (d) {x 3 – x, 2x2 + 4, -2x3 + 3x2 + 2x + 6} in P3(R) (e) {(1, -1, 2), (1, -2, 1), (1, 1, 4)} in R3 (f) {(1, –1, 2), (2, 0, 1), (–1, 2, –1)} in R3 {(÷ ) ( -) (; 0. 2 ).(- )} in Max2(R) (g) (m) {(-; ') (? ). -2)} in M2x2(R) () (x 4 - х3 + 5x2 - 8х + 6, -х4 + х3 - 5х2 + 5х - 3, x 4 + 3x2 – 3x + 5, 2x4 + 3x3 + 4x2 – x + 1, x3 – x + 2} in P4(R) ) (x4 - х3 + 5x2 - 8х + 6, -х4 + x3 - 5x2 + 5х - 3, х4 + 3x2 - 3х + 5, 2х4 + х3 + 4x2 + 8х} in P4(R)
Let H = {(1), (12)(34), (13)(24), (14)(23)}. Find the left cosets of H in A4 (see Table 5.1 on page 111).
3) Let T be a linear transformation from M22 (R) to P3 (R). [ 16 36 36}] Let C = (1 + 1x + 0x² + 0x³), (0+ 1x + 1x² + 0x³), (0+ 0x + 1x² + 1x³), (0+ 0x + 0x² + 1x³). Let B = Let M = 2 -2 0 -3 10 12 -11 0 -14 1 -1 1 -19 2 -25 be the matrix transformation of T from basis B to C. The closed form of the transformation is T a C d =
7) Find the Jacobian matrix at The point (1,2,1.) Of The following Funchun: sudeversh to audingsh F(21,4, 2) (x₁³ + 2y₂ +2²₁ y² + 2xy + y2²)
Let M-{1, 2}. Then O IP(MXM)I=4 None of the mentioned MxM=(1, 4) M×M={(1,1),(2,2)} O IP(MxM)|=16
3) show that (N) = ENONP(N,V) = Nop and (N²) – (N)² = Nop(1– p) hint : write N2 = N(N – 1)+N N=0+ 4) show that if both Nop and No(1– p) are big numbers, the function P(N,V) has a Gaussian form. Where is the center of the gaussian and what is its width? hint : set x – Nop, rewrite log P(N,V) as a function of x and use Stirling's formula. Assume x << Nop and x << No(1- p), expand for small x.
1. Consider two MA(1) models: xt = Wt + 1 2Wt-1, Wt ~ N(0, 2²) Ut ~ Yt = vt +2Vt-1, (a) Why do we prefer invertible models? (b) Check if both models are invertible using the lag operator B. (c) Find the mean functions for both models. (d) Find the autocovariance functions for both models. (e) Find the autocorrelation functions for both models. (f) Simulate 100 observations for each model, and compare the time plots of the simulated series. Set a seed, and use it for both. (g) Which of the two models above is preferred? Give one reason. N(0, 1²)
The functions y1, 22, ..., Yn are linearly independent, if and only if W (y1, 22, ..., Yn)(to) #0 for some to- True False
Consider a labour market with a labour supply function Is i = Bo + B₁W₁ +8² and a labour demand fuction Id = Yo + ed and a market equilibrium condition I = Ld. In i addition E[¹] = E[ed]= 0 and Cov(es, ed=0. eq) a) Show that Cov(Wi, &) f-0. b) Show that the OLS estimator of B₁ is inconsistent.
2.4 (Ross, 2003, page 593) Show that the relationship $(x) = x;¢(1;, x) + (1 – x;)$(0;, x) is true for any coherent structure function o (x) and any i = 1, 2, ., n. ...9
Find x1, x2, x3 with gauss jordan