Solution (incorrect!) (i) Group G: Modular multiplication is commutative, so G is abelian. Hence, by Theorem B35, G is cyclic. In (G, X21) we have: 2² = 4, 2³ = 8, 24 = 16, 25 16 ×21 2 = 11, 26 11 X21 2 = 1. Thus (2) = {1, 2, 4, 8, 11, 16} = G, and hence 2 is a generator for G. Group H: We can see from the table that (e) = {e, r, s, t, u, v}, (s) = {s, e,r, u, v, t}, (u) = {u, v, t, s, e, r}, Thus (H, *) is cyclic, because every element of H is a generator. (ii) (G, X21) and (Z6, +6) are both cyclic groups of order 6, so they are isomorphic and we can find an isomorphism by using Strategy B6. From part (i), 2 is a generator for G. We choose the generator 1 for Z6. This gives the following isomorphism: : (G, X21) → (Z6, +6) 110 2 1 (r) = {r, s, e, v, t, u}, (t) = {t, u, v, e, r, s}, (v) = {v, t, u, r, s, e}. 412 8 3 114 165. (b) Write out a correct solution to the exercise.
Solution (incorrect!) (i) Group G: Modular multiplication is commutative, so G is abelian. Hence, by Theorem B35, G is cyclic. In (G, X21) we have: 2² = 4, 2³ = 8, 24 = 16, 25 16 ×21 2 = 11, 26 11 X21 2 = 1. Thus (2) = {1, 2, 4, 8, 11, 16} = G, and hence 2 is a generator for G. Group H: We can see from the table that (e) = {e, r, s, t, u, v}, (s) = {s, e,r, u, v, t}, (u) = {u, v, t, s, e, r}, Thus (H, *) is cyclic, because every element of H is a generator. (ii) (G, X21) and (Z6, +6) are both cyclic groups of order 6, so they are isomorphic and we can find an isomorphism by using Strategy B6. From part (i), 2 is a generator for G. We choose the generator 1 for Z6. This gives the following isomorphism: : (G, X21) → (Z6, +6) 110 2 1 (r) = {r, s, e, v, t, u}, (t) = {t, u, v, e, r, s}, (v) = {v, t, u, r, s, e}. 412 8 3 114 165. (b) Write out a correct solution to the exercise.
Advanced Engineering Mathematics
10th Edition
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Erwin Kreyszig
Chapter2: Second-order Linear Odes
Section: Chapter Questions
Problem 1RQ
Related questions
Question
The I,I,iii within the exercise are not required as it is an example. A and B are required pleas
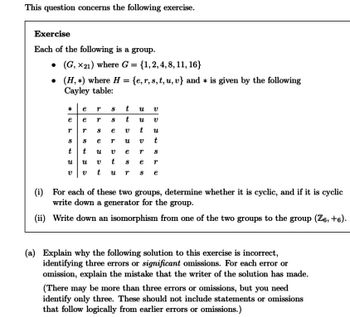
Transcribed Image Text:This question concerns the following exercise.
Exercise
Each of the following is a group.
. (G, X21) where G = {1, 2, 4, 8, 11, 16}
. (H,*) where H = {e, r, s, t, u, v} and is given by the following
Cayley table:
*
e T
e
T
T
S
S
e
t t
U
u
u
V
V
V t
e
T
s
S
S
e
T
5
t U V
t
U
V
V t
U
U V
t
e T
S
S e T
se
t
u T
(i) For each of these two groups, determine whether it is cyclic, and if it is cyclic
write down a generator for the group.
(ii) Write down an isomorphism from one of the two groups to the group (Z6, +6).
(a) Explain why the following solution to this exercise is incorrect,
identifying three errors or significant omissions. For each error or
omission, explain the mistake that the writer of the solution has made.
(There may be more than three errors or omissions, but you need
identify only three. These should not include statements or omissions
that follow logically from earlier errors or omissions.)
Transcribed Image Text:Solution (incorrect!)
(i) Group G:
Modular multiplication is commutative, so G is abelian.
Hence, by Theorem B35, G is cyclic.
In (G, X21) we have:
22 =
2³ = 8,
4,
24 = 16,
25
16 ×21 2 = 11,
26 11 X21 2 = 1.
Thus (2) = {1, 2, 4, 8, 11, 16} = G, and hence 2 is a generator
for G.
Group H:
We can see from the table that
(e) = {e, r, s, t, u, v},
(s) = {s, e,r, u, v, t},
(u) = {u, v,t, s, e, r},
Thus (H, *) is cyclic, because every element of H is a generator.
(ii) (G, X21) and (Z6, +6) are both cyclic groups of order 6, so they are
isomorphic and we can find an isomorphism by using Strategy B6.
From part (i), 2 is a generator for G. We choose the generator 1
for Z6. This gives the following isomorphism:
: (G, X21)→→ (Z6, +6)
1 0
(r) = {r, s, e, v, t, u},
(t) = {t, u, v, e, r, s},
(v) = {v, t, u, r, s, e}.
21
412
83
114
165.
(b) Write out a correct solution to the exercise.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by step
Solved in 4 steps with 4 images
Recommended textbooks for you
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Advanced Engineering Mathematics
Advanced Math
ISBN:
9780470458365
Author:
Erwin Kreyszig
Publisher:
Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:
9780073397924
Author:
Steven C. Chapra Dr., Raymond P. Canale
Publisher:
McGraw-Hill Education
Introductory Mathematics for Engineering Applicat…
Advanced Math
ISBN:
9781118141809
Author:
Nathan Klingbeil
Publisher:
WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:
9781337798310
Author:
Peterson, John.
Publisher:
Cengage Learning,
