
Calculus: Early Transcendentals
8th Edition
ISBN: 9781285741550
Author: James Stewart
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Concept explainers
Question
find the critical values
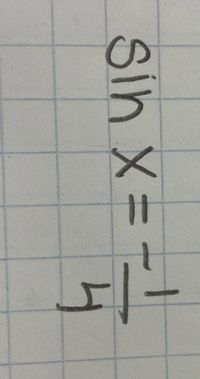
Transcribed Image Text:1.
Sin x = - |
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, calculus and related others by exploring similar questions and additional content below.Similar questions
- Find the linear curve fit coefficients. Use equations (4a) and (4b) to find these coefficients and determine the units of the coefficients, c1 and c2. Note that the units for these coefficients will not be the same in our two cases. Further note that the calibration coefficients are intermediate quantities, not final results. It is probably best to keep them to four significant digits to avoid round-off errors in subsequent calculations. Lastly, plot the line representing the curve fit. It should appear as a best fit, if you’ve done everything correctly. n is the number of data pairs in the curve-fit. the yi ’s arethe ordinate values (the less precisely known). the xi ’s are the abscissa values (the more precisely known).arrow_forwardA chemistry experiment is performed measuring the solubility of potassium chloride (KCl) in water at different temperatures. The goal was to determine if there is a linear relationship between the temperature of the water and how much KCl can dissolve, measured as grams per 100 milliliter (g/100mL). After the experiments were performed, the following data was collected with temperature being the independent x-variable and solubility being the dependent y-variable: Temperature (°C) x Solubility (g/100mL) y 10 31 20 33 30 37 40 41 50 42 Based on the data given for temperature and solubility of KCl and without doing any math yet, which of the following do you predict would best describe the relationship between these variables? A positive linear relationship (r close to 1) A positive linear relationship (r close to -1) A negative linear relationship (r close to 1) A negative linear relationship (r close to -1)…arrow_forwardThe number of banks in a country has been dropping steadily since 1984, and the trend in recent years has been roughly linear. The annual data for the years 1999 through 2008 can be summarized as follows, where x represents the years since 1990 and y the number of banks, in thousands. n=10 Ex-235 ²-5605 Ey-77.564 Ey²=603.80424 Exy-1810.155 a. Find an equation for the least squares line. b. Use your result from part a to predict the number of banks in the year 2020. c. If this trend continues linearly, in what year will the number of banks drop below 6200? d. Find and interpret the correlation coefficient.arrow_forward
- The following chart shows the percentage of the U.S. discretionary budget allocated to education from 2003 to 2009 (t = 3 represents the start of 2003). A bar graph titled "Percentage of U.S. budget on education" has a horizontal t-axis labeled "Year since 2000" and a vertical axis labeled "Percentage". The bar graph has 7 bars. Each bar is associated with a label and an approximate value as listed below. 3: 6.8% 4: 7% 5: 7% 6: 6.9% 7: 6.5% 8: 6.3% 9: 6.2% The following table shows the percentage of the U.S. discretionary budget allocated to education in 2003, 2005, and 2009 (t = 3 represents the start of 2003). Year t 3 5 9 Percentage 6.8 7 6.2 Find a quadratic model for these three years. (Round your coefficients to two decimal places.) p(t) = Use your model to estimate the percentage of the U.S. discretionary budget allocated to education in 2007. (Round your answer to two decimal places.)arrow_forwardI just need the d sectionarrow_forwardI need help with this pleasearrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning
Calculus: Early TranscendentalsCalculusISBN:9781285741550Author:James StewartPublisher:Cengage Learning Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON
Thomas' Calculus (14th Edition)CalculusISBN:9780134438986Author:Joel R. Hass, Christopher E. Heil, Maurice D. WeirPublisher:PEARSON Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON
Calculus: Early Transcendentals (3rd Edition)CalculusISBN:9780134763644Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric SchulzPublisher:PEARSON Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
Calculus: Early TranscendentalsCalculusISBN:9781319050740Author:Jon Rogawski, Colin Adams, Robert FranzosaPublisher:W. H. Freeman
 Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning
Calculus: Early Transcendental FunctionsCalculusISBN:9781337552516Author:Ron Larson, Bruce H. EdwardsPublisher:Cengage Learning

Calculus: Early Transcendentals
Calculus
ISBN:9781285741550
Author:James Stewart
Publisher:Cengage Learning

Thomas' Calculus (14th Edition)
Calculus
ISBN:9780134438986
Author:Joel R. Hass, Christopher E. Heil, Maurice D. Weir
Publisher:PEARSON

Calculus: Early Transcendentals (3rd Edition)
Calculus
ISBN:9780134763644
Author:William L. Briggs, Lyle Cochran, Bernard Gillett, Eric Schulz
Publisher:PEARSON

Calculus: Early Transcendentals
Calculus
ISBN:9781319050740
Author:Jon Rogawski, Colin Adams, Robert Franzosa
Publisher:W. H. Freeman


Calculus: Early Transcendental Functions
Calculus
ISBN:9781337552516
Author:Ron Larson, Bruce H. Edwards
Publisher:Cengage Learning