Related questions
Question
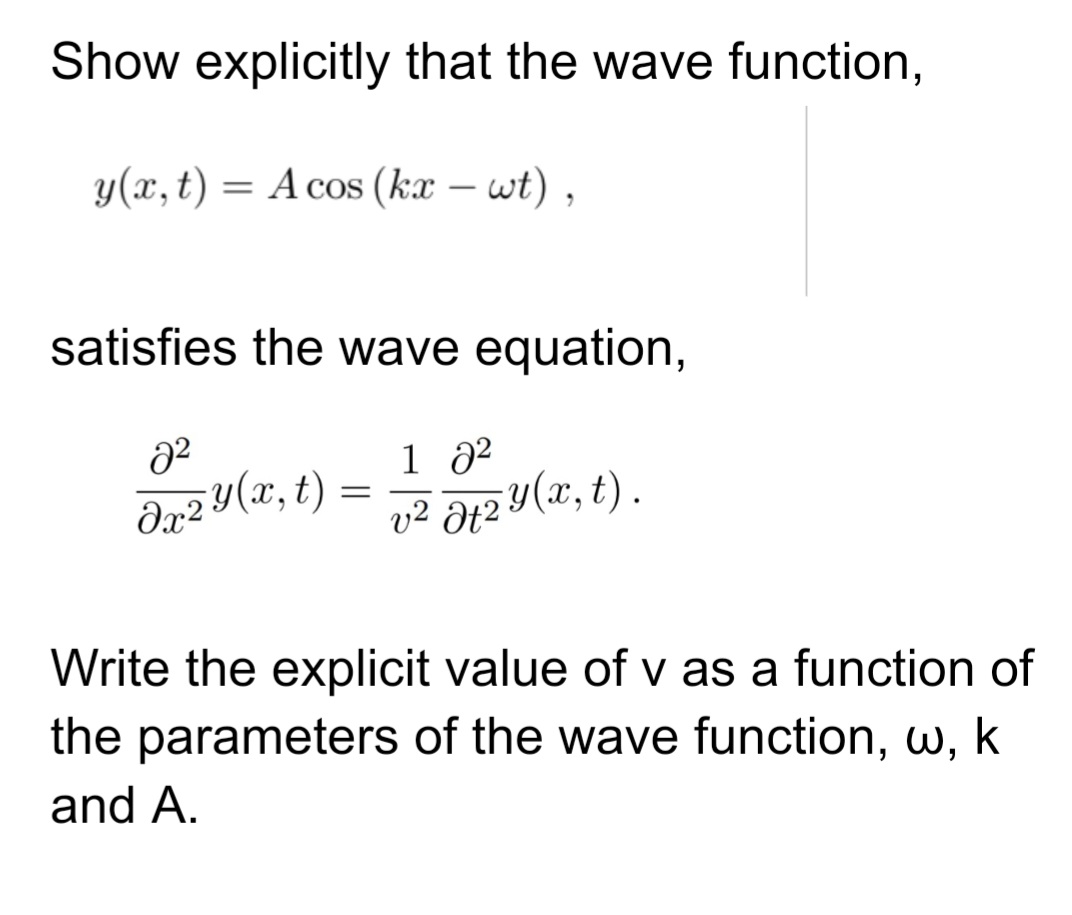
Transcribed Image Text:Show explicitly that the wave function,
y (x, t)
A cos(kx - wt),
=
satisfies the wave equation,
8²
dx 2 y (x, t)
=
1 8²
v² Ət2 y(x, t).
Write the explicit value of v as a function of
the parameters of the wave function, w, k
and A.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps
Knowledge Booster
Similar questions
- a) Show that H = Hoei(wt-kz)az Is a solution to the vector wave equation: 1 a²Ĥ = 0 c2 at2 v2 Η +arrow_forward%90 0 4G VeWIF Two sinusoidal waves of wavelength A = 2/3 m and amplitude A =6 cm and %3D differing with their phase constant, are travelling to the left with same velocity v = 50 m/s. The resultant %3D wave function y_res (x,t) will have the form: y_res (x,t) = 12(cm) cos(p/2) sin(150tx-3nt+p/2). y_res (x,t) = 12(cm) cos(p/2) sin(3rx-180rnt+p/2). %3D y_res (x,t) = 12(cm) cos(9/2) sin (3πx+150 πt+φ/2). y_res (x,t) = 12(cm) cos(p/2) sin(3tx-150rt+p/2). y_res (x,t) = 12(cm) cos(p/2) sin(150Ttx+3nt+p/2). %3Darrow_forwardA standing wave us given by y(x,t) = 10cos(5(pi)t)*sin((2/3)(pi)x) find two waves that could be superimposed to generate this standing wave.arrow_forward
- Consider a point source emitting spherical waves equally in all directions. The amplitude of the wave varies inversely with r, the distance from the point source. The energy flux vary like: O 1/r^2 O Is independent of r r r^2 1/r O 1/r^3 O O O O OOarrow_forwardI need the answer as soon as possiblearrow_forwardSimple hormic motionarrow_forward
- Examine the wave function below. Suppose there is a point that goes from y = 2 mm to y = 6 mm. How long does it take for the point to make the move?arrow_forwardShow by direct substitution that the exponential Gaussian function defined by ?(x,t) = ae-(bx-ct)^2 satisfies the wave equation: (?2?(?,?))/(?x2) = (1/v2) * (?2?(x,t))/(?t2) if the wave is given by the v = (c/b) and a, b, and c are constants.arrow_forwardGive me a correct solution of the sup-part (A), no handwrittenarrow_forward
- a pulse traveling along a string of linear mass density μ as a wave function, y(x), if the power carried by this wave at a point x is described as P(x): P (x) = (μ ω3 / k) e πx 1) What is the power P(0) carried by this wave at the origin? 2) Compute the ratio P(x)/P(0).arrow_forwardFor the nonsinusoidal wave, suppose that E = 100 V/m = 100 N/C. Find the value of B, the energy density u, and the rate of energy flow per unit area Sarrow_forwardProve that the following function f(x,t)=ABe-B(x-vt) satisfies the wave equation 2yot2=v2a2yax2 *arrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios