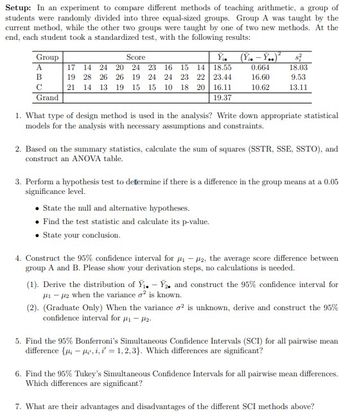
Setup: In an experiment to compare different methods of teaching arithmetic, a group ofstudents were randomly divided into three equal-sized groups. Group A was taught by thecurrent method, while the other two groups were taught by one of two new methods. At theend, each student took a standardized test, with the following results:GroupABCGrandScoreY₁.17 14 24 20 24 23 16 15 14 18.551928 26 26 19 24 24 23 22 23.4421 14 13 19 15 15 10 18 20 16.1119.37(Y₁.-Y..)²0.66416.6010.6218.039.5313.111. What type of design method is used in the analysis? Write down appropriate statisticalmodels for the analysis with necessary assumptions and constraints.2. Based on the summary statistics, calculate the sum of squares (SSTR, SSE, SSTO), andconstruct an ANOVA table.State the null and alternative hypotheses.Find the test statistic and calculate its p-value.State your conclusion.3. Perform a hypothesis test to determine if there is a difference in the group means at a 0.05significance level.4. Construct the 95% confidence interval for ₁-2, the average score difference betweengroup A and B. Please show your derivation steps, no calculations is needed.(1). Derive the distribution of Y₁.-Y. and construct the 95% confidence interval for1-2 when the variance o² is known.(2). (Graduate Only) When the variance o2 is unknown, derive and construct the 95%confidence interval for 1-₂-5. Find the 95% Bonferroni's Simultaneous Confidence Intervals (SCI) for all pairwise meandifference {μμ,i,i=1,2,3). Which differences are significant?6. Find the 95% Tukey's Simultaneous Confidence Intervals for all pairwise mean differences.Which differences are significant?7. What are their advantages and disadvantages of the different SCI methods above?

Since you have posted a question with multiple subparts, we will provide solution to the first three sub-parts as per our Q&A guidelines. Please repost the remaining sub parts separately.The design model used in the study was completely randomized design (CRD) specifically a one-way analysis of variance (ANOVA) design, to compare the means of three or more groups to determine whether there are statistically significant differences among them. The model can be given as: where Yij is the jth observation in ith group, is the overall/grand mean, is the effect of ith group and eij is the error term. Assumptions and Constraints:We assume that the test scores of students in each group are independent of each other.We assume that the errors are normally distributed..We assume that the variances of the test scores within each group are approximately equal.SSTR (treatment sum of squares)= = 250. 956SSTO (total sum of squares) = = (17-19.37)2+(14-19.37)2+(24-19.37)2+.................................+(18-19.37)2+(20-19.37)2 = 576.296SSE (error sum of squares) = SSTO - SSTR = 576.296 - 250.956 = 325.340Treatment degrees of freedom (DF) = number of groups - 1 = 3-1 = 2Error degrees of freedom = Total sample size - number of groups = 9*3 - 3 = 27-3 = 24Total degrees of freedom = 24 + 2 = 26ANOVA table:SourceSSDFMSF-valueTreatment250.9562125.4789.256Error325.3402413.55583Total576.29626MS = SS/DF and F = MS(TR)/MS(E)Null hypothesis: There are no significant differences in mean test scores among the three groups.Alternative hypothesis: The mean test score of at least one group mean is significantly different from the others.Given 0.05 significance level.Test statistic, F = 9.256 (from ANOVA table).P-value = Pr(F>9.256) = 0.00092 (from R software).Since the p-value < 0.05, we reject the null hypothesis. We can conclude that the mean test score of at least one group mean is significantly different from the others.R-codes:1> pf(9.256, df1=2, df2=26, lower.tail = FALSE) #p-value 2[1] 0.00092Answers are given above.