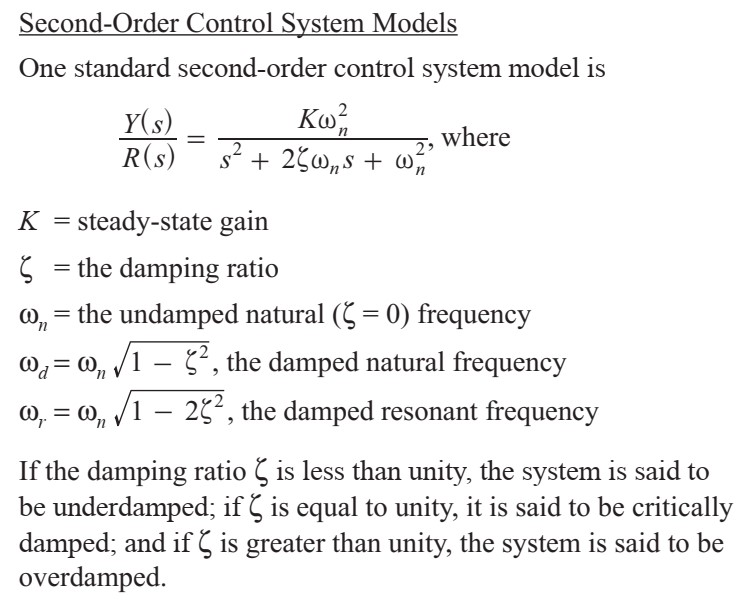
Second-Order Control System Models One standard second-order control system model is Ко 2 3 Y(s) R(s) where п K steady-state gain the damping ratio the undamped natural ( 0) frequency , the damped natural frequency о, 1 o 125, the damped resonant frequency If the damping ratio is less than unity, the system is said to be underdamped; if is equal to unity, it is said to be critically damped, and if Ç is greater than unity, the system is said to be overdamped 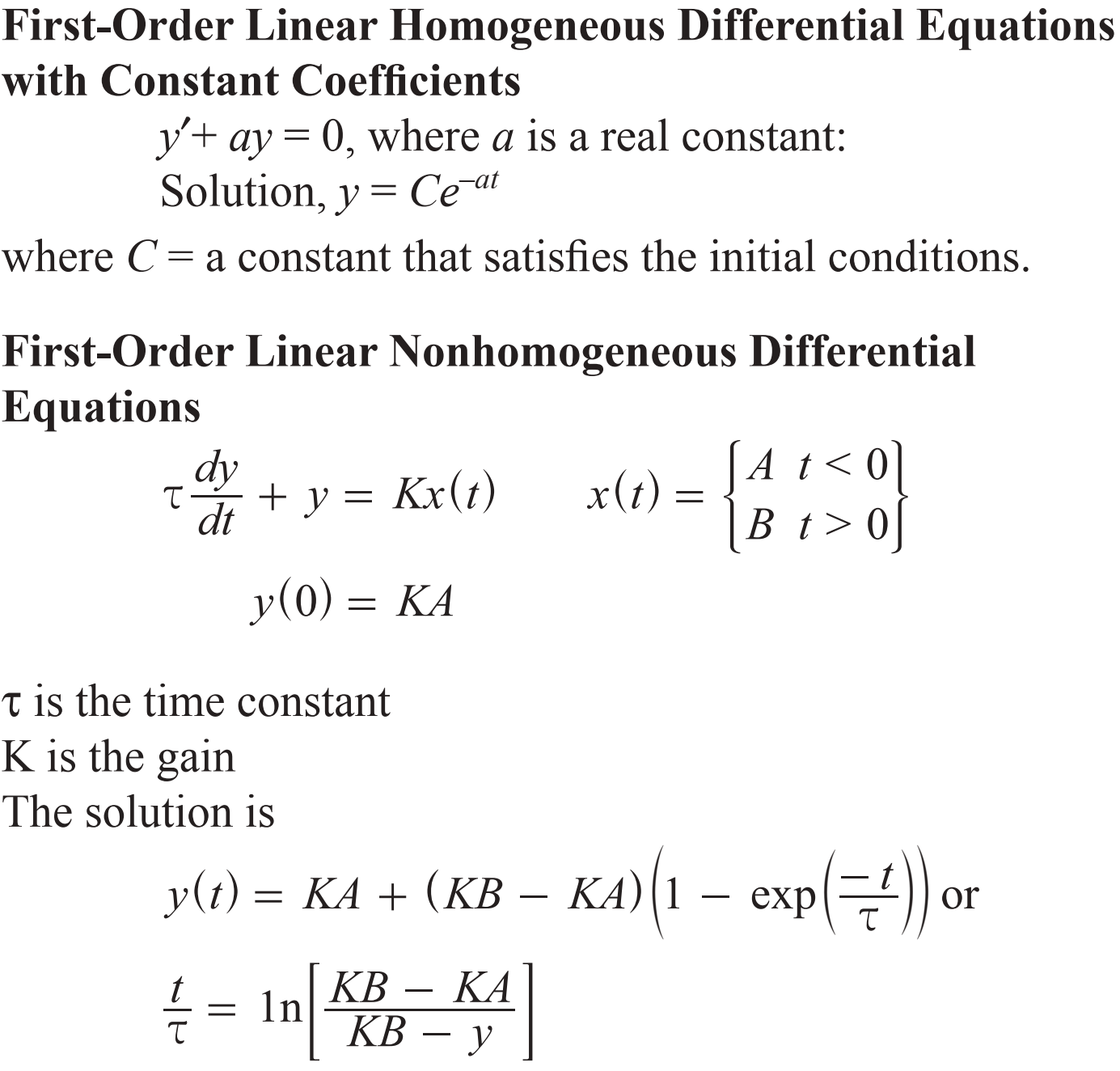First-Order Linear Homogeneous Differential Equations with Constant Coefficients yay Solution, y a constant that satisfies the initial conditions 0, where a is a real constant: Ce a where C First-Order Linear Nonhomogeneous Differential Equations 1 < 0l dy x(t) = + y = Kr(t) |B t> of dt y(0) KA t is the time constant K is the gain The solution is KA (KB KA)1 - exp t or y(t) = In КА КВ t КВ

Have you ever observed the movement of the satellites and rockets or how the planets go around the sun in their orbits? How will you determine their motion? Definitely they follow some scientific laws and these kinds of problems are framed as differentia…