
Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Question
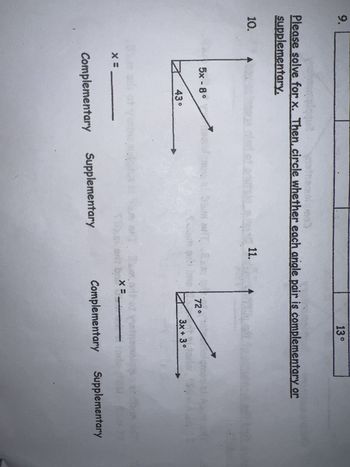
Transcribed Image Text:### Solving for \( x \) in Angle Pair Problems
Please solve for \( x \). Then circle whether each angle pair is complementary or supplementary.
#### Problem 10:
Given angles:
- \( 5x - 8^\circ \)
- \( 43^\circ \)
Diagram:
- The two angles form a right angle (90 degrees).
Solution steps:
1. Determine the equation based on the sum of complementary angles:
\( (5x - 8^\circ) + 43^\circ = 90^\circ \)
2. Simplify and solve for \( x \):
\( 5x - 8 + 43 = 90 \)
\( 5x + 35 = 90 \)
\( 5x = 55 \)
\( x = 11 \)
Verification:
- Substitute \( x \) back into the angle equation:
\( 5(11) - 8 = 55 - 8 = 47 \)
- Check if the angles sum to 90 degrees:
\( 47^\circ + 43^\circ = 90^\circ \)
Conclusion:
- The angle pair is **complementary**.
---
#### Problem 11:
Given angles:
- \( 72^\circ \)
- \( 3x + 3^\circ \)
Diagram:
- The two angles form a right angle (90 degrees).
Solution steps:
1. Determine the equation based on the sum of complementary angles:
\( 72^\circ + (3x + 3^\circ) = 90^\circ \)
2. Simplify and solve for \( x \):
\( 72 + 3x + 3 = 90 \)
\( 3x + 75 = 90 \)
\( 3x = 15 \)
\( x = 5 \)
Verification:
- Substitute \( x \) back into the angle equation:
\( 3(5) + 3 = 15 + 3 = 18 \)
- Check if the angles sum to 90 degrees:
\( 72^\circ + 18^\circ = 90^\circ \)
Conclusion:
- The angle pair is **complementary**.
### Angle Types:
- **Complementary Angles**: Two angles whose measures sum up to 90 degrees.
- **Supplementary Angles**: Two angles whose measures
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning