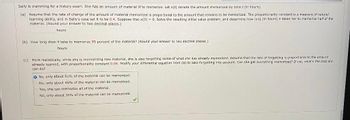
Sally is cramming for a history exam. She has an amount of material M to memorize. Let x(t) denote the amount memorized by time t (in hours). (a) Assume that the rate of change of the amount of material memorized is proportional to the amount that remains to be memorized. The proportionality constant is a measure of natural learning ability, and in Sally's case set it to be 0.4. Suppose that x(0) = 0. Solve the resulting initial value problem, and determine how long (in hours) it takes her to memorize half of the material. (Round your answer to two decimal places.) hours (b) How long does it take to memorize 95 percent of the material? (Round your answer to two decimal places.) hours (c) More realistically, while she is memorizing new material, she is also forgetting some of what she has already memorized. Assume that the rate of forgetting is proportional to the amount already learned, with proportionality constant 0.04. Modify your differential equation from (a) to take forgetting into account. Can she get everything memorized? If not, what's the best she can do? O No, only about 91% of the material can be memorized. No, only about 45% of the material can be memorized. Yes, she can memorize all of the material. No, only about 30% of the material can be memorized.

The population of bears in a national forest is changing according to the function N(t) = 850(0.96)', where t is the time in years and N() is the number of bears. Which statement explains how the bear population is changing? A.O The population is decreasing by 4% per year. B. The population is increasing by 96% per year. c.O The population is decreasing by 96% per year. D.O The population is increasing by 4% per year.