Question
Please as soon as possible
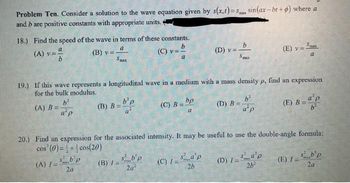
Transcribed Image Text:Problem Ten. Consider a solution to the wave equation given by s(x,t)=sm sin(ax-bt+) where a
and bare positive constants with appropriate units. -
a
18.) Find the speed of the wave in terms of these constants.
(A) v = a
Smar
(B) v =
(A) /-b³p
2a
2/0
(B) B-bp
(C) v==
a
(B) /=
19.) If this wave represents a longitudinal wave in a medium with a mass density p, find an expression
for the bulk modulus.
(A) B=
b²
a²p
s b'p
2a²
bp
a
(C) B=b
(C) /=
(D) v=-
S
sa'p
2b
b
(D) B=
max
20.) Find an expression for the associated intensity. It may be useful to use the double-angle formula:
cos' (0) = + cos(20)
(D) /=
a p
(E) v=
sa p
26²
a
(E) B-p
b²
(E) I=
s b'p
2a
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
arrow_back_ios
arrow_forward_ios