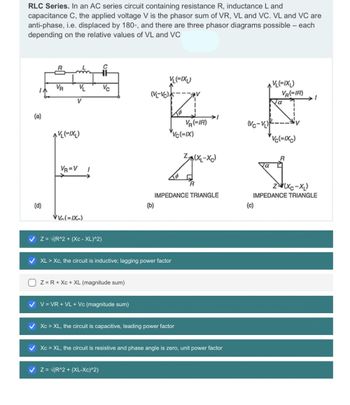
RLC Series. In an AC series circuit containing resistance R, inductance L and capacitance C, the applied voltage V is the phasor sum of VR, VL and VC. VL and VC are anti-phase, i.e. displaced by 180°, and there are three phasor diagrams possible - each depending on the relative values of VL and VC (a) (d) R VR V V (=IX₂) VR=V VV (=IX) 1 ✓ Z= √(R^2 + (XC-XL)^2) Vc Z = R + Xc + XL (magnitude sum) VL(=IXL) (V-VC) (b) ✔ XL > Xc, the circuit is inductive; lagging power factor To av VR (=IR) VC(=IX) Z(XL-XC) IMPEDANCE TRIANGLE (Vc-V₁) VL(=IXL) VR(-IR) (c) Ja iv Vc(=IXC) (Xc−X) IMPEDANCE TRIANGLE

6) Build an Arduino code that has keypad, LCD display. In this code you are required to: (Void Setup and Loop only) 1- Show "Hello Ardunio" blinking on LCD display with delay 1sec when pushing key I from the keypad and the LED is OFF. 2- Make a LED blinking with delay 1sec when pushing key 2 from the keypad and the LCD display is empty 7)An image of 250x250 elements (pixels) what is the memory size of the image if: a)Unit 8 b) Unit 16 c) Black & Whi 8) The game port register is used for two different purposes that are: 1- 2- 9) What is the FPGA device? 10) chose the correct answer for the following: 1- What is a result of connecting two or more switches together? a) The size of the broadcast domain is increased. b) The number of collision domains is reduced. b) The size of the collision domain is increased. c)The number of broadcast domains is increased.

Messages AA Statement one Training will allow you to safely erect a mobile. tower scaffold on any ground surface condition. and access the tower both internal and externally Statement two In windy conditions placing sheeting around the mobile tower scaffold will protect the workers on the tower a) Statement two is incorrect b) Statement one and two are correct c) Statement one is correct and statement two is incorrect d) Both statements are incorrect actclass.co.uk 22 Questi 23 Questi 24 Questi 25 Questi 26 Questi Submit Answers Ć

Fill in the blanks. I would love to see how to get the correct answers.

Correct and detailed answer will be Upvoted else downvoted. Thank you!

Please send the answer by typing only. I don't want handwritten.

Note:Please do not handwritten. Also, please write a lot of information in your own words.

Can you solve in detail? Other wise ready for Downvote. And not give copy paste other or online platform. explain type of electronic material in detail.

18: A solar cooker uses hot air to cook food. Select one: True False 19: text _____________ can be produced by fermentation of animal dung and green leaves. a. Wet process b. Biogas c. MSW d. Land Fill

A galvanometer is used as a _________a) current sourceb) voltage sourcec) null detectord) input impedance