
Trigonometry (MindTap Course List)
10th Edition
ISBN: 9781337278461
Author: Ron Larson
Publisher: Cengage Learning
expand_more
expand_more
format_list_bulleted
Question
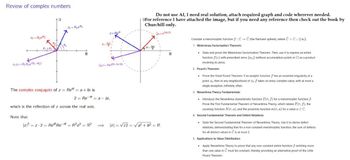
Transcribed Image Text:Review of complex numbers
1=R₁e
z=Re
Do not use AI, I need real solution, attach required graph and code wherever needed.
For reference I have attached the image, but if you need any reference then check out the book by
Churchill only.
Ca-e2/8
22=
R₂e
2122=R₁ Re(+₂)
01+02
R
Saz-Re40+2x/8)
The complex conjugate of z = Rei = a + bi is
z= Rea-bi.
which is the reflection of z across the real axis.
Note that
Iz²=z z=Re Re-i = R2e0 = R²
=>
Consider a meromorphic function f : C+C (the Riemann sphere), where C = CU{0}.
1. Weierstrass Factorization Theorem:
State and prove the Weierstrass Factorization Theorem. Then, use it to express an entire
function f(x) with prescribed zeros {a} (without accumulation points in C) as a product
involving its zeros.
2. Picard's Theorem:
Prove the Great Picard Theorem: If an analytic function f has an essential singularity at a
point zo, then in any neighborhood of zu, f takes on every complex value, with at most a
single exception, infinitely often.
3. Nevanlinna Theory Fundamentals:
Introduce the Nevanlinna characteristic function T(r, f) for a meromorphic function f.
Prove the First Fundamental Theorem of Nevanlinna Theory, which relates T(r. f), the
counting function N(r,a), and the proximity function m(r, a) for a value a € C.
4. Second Fundamental Theorem and Defect Relations:
State the Second Fundamental Theorem of Nevanlinna Theory. Use it to derive defect
relations, demonstrating that for a non-constant meromorphic function, the sum of defects
for all distinct values in C is at most 2.
5. Applications to Value Distribution:
Apply Nevanlinna Theory to prove that any non-constant entire function f omitting more
than one value in Ĉ must be constant, thereby providing an alternative proof of the Little
Picard Theorem.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 6 images

Knowledge Booster
Similar questions
- Review of complex numbers 1=R₁e 22-R₂e 2122=R₁ Re(+82). z=Re Do not use AI, I need real solution, attach required graph and code wherever needed. 3For reference I have attached the image, but if you need any reference then check out the book by Churchill only. Caz-Re(+2x/8) The complex conjugate of z = Rei = a + bi is z=Rea-bi which is the reflection of z across the real axis. Note that Iz zz Re Re = R²e = R² z=√√zz=√√a²+ b² = R. Consider a harmonic function u: D→ R. where DCC is an open connected domain. 1. Harmonic Conjugates: a. Prove that if u is harmonic on D and simply connected, then there exists a harmonic conjugate such that fu+ie is holomorphic on D. ⚫b. Provide an explicit construction of given u in a specific example, such as u(x, y) = 22-y². 2. Maximum Principle for Harmonic Functions: ⚫a. State and prove the Maximum Principle for harmonic functions, asserting that if u attains its maximum (or minimum) value in the interior of D, then u is constant. b. Apply the Maximum…arrow_forwardANSWER A AND B FROM PROB 4 SHOW STEPS OF COMPLEX NUMBER IN RECTANGULAR FORMarrow_forwardSimplify the complex number (Z^3) * ?and find the Re(z) & Im(z)arrow_forward
- Review of complex numbers =Rje 22=R₂e z=Re 2122 R1 R₂+82) Caz-Re(+2x/8) The complex conjugate of z = Rei=a+bi is z=Re-a-bi, which is the reflection of z across the real axis. Note that Do not use AI, I need real solution, attach required graph and code wherever needed. For reference I have attached the image, but if you need any reference then check out the book by Churchill only. Ca-e2/8 |z2=zz Re Re = R2e0 = R2 => |z|= √√zz = √√a² + b² = R. Problem 5: The Cauchy Integral Formula and Its Consequences Statement: Let D be a bounded, simply connected domain in C with a piecewise smooth boundary D, and let f: D→ C be holomorphic on D and continuous on D. 1. Cauchy Integral Formula: Prove the Cauchy Integral Formula: For any z € D, 2. Cauchy's Estimates: f(C) f(z) = 2mi Using the Cauchy Integral Formula, derive Cauchy's estimates for the derivatives of f. Specifically, show that for any n≥ 0, |f(") (=)|≤ n!M R where M = maxcap |f(C) and R is the distance from z to OD. 3. Taylor and Laurent…arrow_forwardI need it solved for preparation to tomorrow exam help Please ! ? I have answers in the system without step by step help !arrow_forwardReview of complex numbers Do not use AI, I need real solution, attach required graph and code wherever needed. For reference I have attached the image, but if you need any reference then check out the book by Churchill only. Z2=R2e2 21=R1e01 z=Reia (8=e²xi/8 01+02 R R Z122 R1 R2e1+82) $82=Rei(+2x/8) The complex conjugate of z = Reie = a + bi is Z= Rea- bi, which is the reflection of z across the real axis. Note that |z2z-Z Re Re-i = R²e = R² => |z|= √√√zz = √√√a² + b² = R. Let D and D' be simply connected domains in C, and let f : DD' be a biholomorphic (conformal) map. 1. Preservation of Laplace's Equation: Show that if u : D'→R is a harmonic function on D', then u of is a harmonic function on D. 2. Conformal Invariance of the Laplacian: • Prove that the Laplacian operator is conformally invariant up to a scaling factor. Specifically, demonstrate that for f: DD' biholomorphic and u twice continuously differentiable, A(uf) = f(z)|(Au) of. 3. Dirichlet Problem and Conformal Mapping: ⚫…arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage
Trigonometry (MindTap Course List)TrigonometryISBN:9781337278461Author:Ron LarsonPublisher:Cengage LearningAlgebra & Trigonometry with Analytic GeometryAlgebraISBN:9781133382119Author:SwokowskiPublisher:Cengage Intermediate AlgebraAlgebraISBN:9781285195728Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning
Intermediate AlgebraAlgebraISBN:9781285195728Author:Jerome E. Kaufmann, Karen L. SchwittersPublisher:Cengage Learning

Trigonometry (MindTap Course List)
Trigonometry
ISBN:9781337278461
Author:Ron Larson
Publisher:Cengage Learning

Algebra & Trigonometry with Analytic Geometry
Algebra
ISBN:9781133382119
Author:Swokowski
Publisher:Cengage

Intermediate Algebra
Algebra
ISBN:9781285195728
Author:Jerome E. Kaufmann, Karen L. Schwitters
Publisher:Cengage Learning