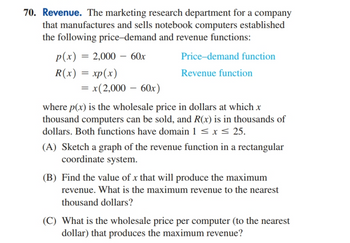
Revenue. The marketing research department for a company that manufactures and sells notebook computers established the following price-demand and revenue functions: p(x) R(x) = xp(x) = 2,000 - 60x = x(2,000 - 60x) Price-demand function Revenue function where p(x) is the wholesale price in dollars at which x thousand computers can be sold, and R(x) is in thousands of dollars. Both functions have domain 1 ≤ x ≤ 25. (A) Sketch a graph of the revenue function in a rectangular coordinate system. (B) Find the value of x that will produce the maximum revenue. What is the maximum revenue to the nearest thousand dollars? (C) What is the wholesale price per computer (to the nearest dollar) that produces the maximum revenue?

bThe average rate of change of the linear function f(x)=3x+5 between any two points is ________.

A company determines that its weekly profit from manufacturing and selling x units of a certain item is given by Px=-x3+3x2+2880x-500. Use a graphing utility to find the weekly production rate that will maximize the profit.