Rank the speeds of the second block after the collision for each trial from greatest to least in the spaces indicated below. Use “1” for the collision with the largest speed, “2” for the next largest speed, and “3” for the slowest. If any two or all three are the same, use the same number for their ranking.
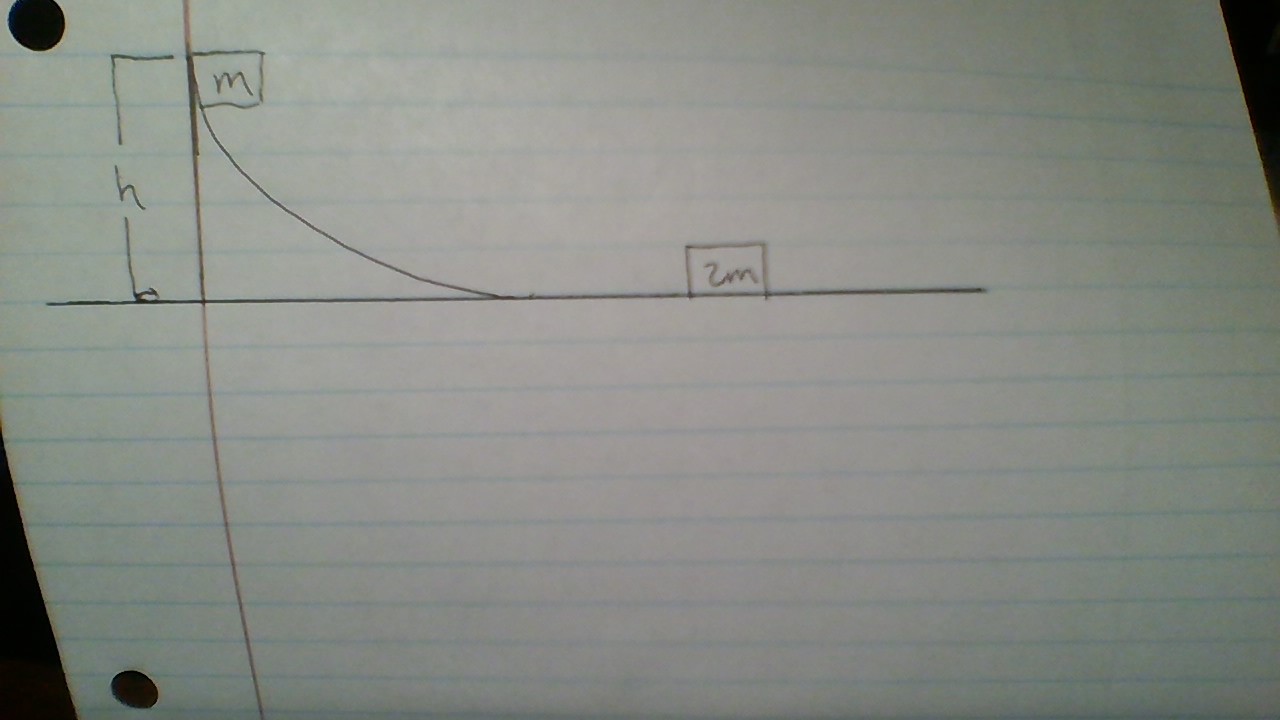
1) The figure above shows part of a system consisting of a block at the top of a ramp secured to a horizontal table, which is located on Earth. If the block with a mass of m slides down a ramp with negligible friction, and if the center of mass of the block decreases its height by h, derive an equation that determines the speed of the block when it first contacts the table.
2) As the first block moves across the table with negligible friction, it contacts a second block, originally at rest, with a mass twice that of the first. Three trials are
a. In the first trial a collision occurs that results in the first block coming to rest.
b. In the second trial a small piece of Velcro, whose mass is insignificant compared to the masses of each block, is added to each block. A collision occurs, the blocks stick and move together as one.
c. In the third trial a small sheet of elastic material, whose mass is insignificant compared to the masses of each block, is added to each block. A collision occurs and the first block bounces off the second block. This collision results in the first block moving to the left and the second block moving to the right.
Rank the speeds of the second block after the collision for each trial from greatest to least in the spaces indicated below. Use “1” for the collision with the largest speed, “2” for the next largest speed, and “3” for the slowest. If any two or all three are the same, use the same number for their ranking. (1 pt.)
_____ a, _____ b, _____ c
In the space provided, justify your answer.
3) In terms of the variables used to describe the speed of the first block in Question #1, determine the speed after the collision described in Question 2.b. when the first block sticks to the second block.
4) The first trial described in Question #2 a. is repeated but an unstretched spring is secured to far right side of the table in the direct path of the second block. The second block strikes the spring and compresses it a distance of x before coming to rest for an instant. Sketch a graph with the distance the spring is compressed on the horizontal axis and the force of the spring on the block on the vertical axis. Below the graph identify what a) the slope of the graph and b) the area between curve and the horizontal axis represent
5) A second ramp, with the same shape and height as the first, is placed next to the first ramp and secured to the table. The same block (with mass m) slides down the first ramp and a solid steel sphere (with a mass of m and radius R) rolls down the ramp without slipping.
a. Which would travel a greater horizontal distance off the end of the table? Justify your answer in a clear, coherent, paragraph-length explanation.
b. Determine the speed of the ball when it reaches the bottom of the ramp in terms of m, R, g, and h. The moment of inertia for a solid sphere is 2/5 mR2 and ω =v/R.
6) In a small paragraph explain how the motion of the block with a mass of m would be different in Question #1 if the ramp (with a mass of 10m) was no longer secured to the frictionless table. Additionally, describe an experiment, using equipment commonly available in a Physics Lab, that can be used to validate your prediction.
Trending now
This is a popular solution!
Step by step
Solved in 4 steps with 6 images
