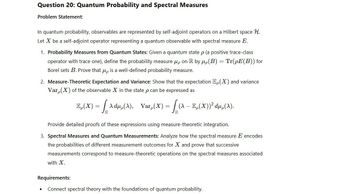
Question 20: Quantum Probability and Spectral Measures Problem Statement: In quantum probability, observables are represented by self-adjoint operators on a Hilbert space H. Let X be a self-adjoint operator representing a quantum observable with spectral measure E. 1. Probability Measures from Quantum States: Given a quantum state p (a positive trace-class operator with trace one), define the probability measure μp on R by μ(B) = Tr(pE(B)) for Borel sets B. Prove that up is a well-defined probability measure. 2. Measure-Theoretic Expectation and Variance: Show that the expectation E(X) and variance Var, (X) of the observable X in the state p can be expressed as E(X) = [Adµ(A), Var(x) = [ {A – E‚(X))² dµ‚(A). Provide detailed proofs of these expressions using measure-theoretic integration. 3. Spectral Measures and Quantum Measurements: Analyze how the spectral measure E encodes the probabilities of different measurement outcomes for X and prove that successive measurements correspond to measure-theoretic operations on the spectral measures associated with X. Requirements: Connect spectral theory with the foundations of quantum probability.

Q. 8.(a) Let {e} be an orthonormal set in a Hilbert space H, then Σ|(x,e₁) | ² ≤||x||² for each vector x in H.

Determine discrete numeric function corresponding to the following generating function: A(Z)=1/1-Z³

7. What is the z-transform of the signal x(n) = -anu(-n-1)? A. B. C. D. 1 1-az -;ROC |z||a| ROC |z|<|al|

What exactly is the Modulus operator?

Let o denote the Euler phi-function. If F is defined by F(n) = Σφ(d?) d|n Is F multiplicative? Explain your answer. L

Let I be the linear operator on R³ defined by L( Find the matrix A representing L with respect to S= Find the matrix B representing L with respect to T= s={ T= ) Find an invertible matrix P such that B=P-¹AP. Turkçesic L, R² üzerinde L( 12 311-12. 1] tabanına göre L nin temsil matrisi A yı bulunuz. O O 5 -1₁ B = P¹AP sağlayan tersi olan P matrisini bulunuz. A= A = 1-15, -3 A L 11 -3 ← 4-11171 A- 9 %], 9 tabanına göre L nin temsil matrisi B yi bulunuz. B = 11 3 ,B 1 18 3- [1/ 18 -7 -12 = +1([2] B 1/2 9/2 1/2 -[24]. B = [ 0 = 1/2 1/2 -3/2] 18 - A.B {/₂2]· 7 1/2 1/2 21 2² 2], P = [¹/² ¹/²] -3/2) 0 1 9/2 371-7₂2 şeklinde tanımlanan bir lineer dönüşüm olsun: 2 [1²7], P = [2²3] P = - [29 [23] P=[ A] -3 ³], P = [1, 2] 2

In Example 3 of Section 3.1, find all elements a of S(A) such that a2=e. From Example 3 of section 3.1: A=1,2,3 and S(A) is a set of bijective functions defined on A.

Calculus Define T:P4P3 by T(p)=p. What is the kernel of T?

6. In Example 3 of section 3.1, find elements and of such that but . From Example 3 of section 3.1: and is a set of bijective functions defined on .