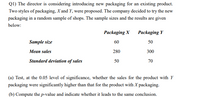
Q1) The director is considering introducing new packaging for an existing product.Two styles of packaging, X and Y, were proposed. The company decided to try the newpackaging in a random sample of shops. The sample sizes and the results are givenbelow:Packaging XPackaging YSample size6050Mean sales280300Standard deviation of sales5070(a) Test, at the 0.05 level of significance, whether the sales for the product with Ypackaging were significantly higher than that for the product with X packaging.(b) Compute the p-value and indicate whether it leads to the same conclusion.

Answered: Q1) The director is considering…

A First Course in Probability (10th Edition)

10th Edition

ISBN:9780134753119

Author:Sheldon Ross

Publisher:Sheldon Ross

Chapter1: Combinatorial Analysis

Section: Chapter Questions

Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...

See similar textbooks

Similar questions

Job satisfaction as a function of a work schedule was investigated in different factories. In the first factory, the employees are on fixed shift system while in the second factor, the workers have rotating shift system. Using the data in the table below, determine if there is a significant difference in job satisfaction between the two groups of workers. Use 0.01 level of significance. Shift Schedule Mean Standard Deviation Sample size Fixed 7.43 2.42 23 Rotating 6.18 2.15 29
Buyer's Digest is rating thermostats manufactured by two manufacturers, Thermorite and Tempking. In a recent test, 28 thermostats from Thermorite and 12 thermostats from Tempking were randomly selected and placed in a test room that was maintained at a temperature of 68°F. Average temperature readings were as follows: Thermorite: 68 Tempking: 66 Sample standard deviations were: Thermorite: 0.52 Tempking: 0.8 If we want to conduct a hypotheses test with a-05 to see whether the temperature reading variance for Thermorite is smaller than Tempking. what is the correct test statistic? (Round your answer to three decimal places) Test Statistic
The president of an all-female school wants to know if the students at her school studied more or less, on average, than the students at a neighboring all-male school. To find out, she conducted a study. Accordingly, samples were taken at each school with the following results: All female: sample size = 43, mean study time ( hours) = 18.56, standard deviation (hours) = 4.35 All male: sample size = 38, mean study time (hours) =17.95, standard deviation (hours) = 4.87 Calculate the 95% confidence interval for the differences between population means. Use brackets write the final answer [x.xx, x.xx]
Q.1.1 Consumer testing services compared public opinion on gas ovens and electric ovens. They asked participants to rate their opinions using a scale from 1 to 10. A random sample of 220 households were interviewed. The descriptive statistics are as follows: MeanStandard deviation Sample size respectively Gas Ovens mean7.45 standars deviation3.32 sample size220 Electric Ovens mean8.85 standard deviation4.14 sample size220 Q.1.1.1 Calculate the standard deviation of the difference between the mean opinion scores of gas ovens and electric ovens. Q.1.1.2 The analyst concluded that there was 50% probability that the sample mean of opinion scores of gas ovens is smaller than that of electric ovens. He used z-transformations and got z = -3.9. Calculate the z value using information in the table to check if the value of -3.9 used by the analyst was correct.
In an effort to determine which company between Primacom Outsourcing Company and Technica Global Services has better customer service, an independent survey group gathered data on the length of time (in minutes) a customer has to wait on hold before someone answers the phone when calling the customer care hotline for assistance. The data is summarized below. Statistics Primacom Outsourcing Company Technica Global Services Mean 8.14 9.426 Standard Deviation 1.345 1.813 Sample Size 7 7 Is there a significant difference between the average lengths of time customers are on hold for the two companies? Use a 5% level of significance. Assume that the lengths of time on hold are normally distributed with unknown but equal population variances. A. Perform a complete test of hypothesis. B. Select here the conclusion for this test of hypothesis. There is a significant difference between the average lengths of time customers are on hold for the two companies. There is no significant difference…
A FOOD PROCESSOR WANTS TO COMPARE TWO PRESERVATIVES FOR THEIR EFFECTS ON RETARDING SPOILAGE. SUPPOSE 16 CUTS OF FRESH MEAT ARE TREATED WITH PRESERVATIVE A AND 16 ARE TREATED WITH PRESERVATIVE B, AND THE NUMBER OF HOURS UNTIL SPOILAGE BEGINS IS RECORDED FOR EACH OF THE 32 CUTS OF MEAT. THE RESULTS ARE SUMMARIZED IN THE TABLE BELOW 4.1 4.2 4.3 Sample Mean Sample Standard Deviation Preservative A 108.7 hours t: 10.5 hours Preservative B 98.7 hours 13.6 hours State the null and alternative hypotheses to determine if the average number of hours until spoilage begins differs for the preservatives A and B. Assume population variances are equal. Calculate the pooled variance and the value of the test statistic. Determine the rejection region at a= .05 and write the proper conclusion. Rejection region: Decision: Conclusion:
B. The department of labor in a state wanted to find the compensation of hotel employees. A random sample of 20 cleaning persons produced the mean hourly earnings of $10.60 with a standard deviation of $1.05. A random sample of 25 bellhops gave the mean hourly earnings of $11.20 with a standard deviation of $1.30. Assume that the hourly earnings of both groups are normally distributed with equal but unknown population variances. Using the 5% significance level, can you conclude that the mean hourly earnings of both groups are not the same in this state?
An industrial plant wants to determine which of two types of fuel, electric or gas, is more cost efficient (measured in cost per unit of energy). Independent random samples were taken of plants using electricity and plants using gas. These samples consisted of 14 plants using electricity, which had a mean cost per unit of $55.06 and standard deviation of $8.25, and 12 plants using gas, which had a mean of $57.80 and standard deviation of $7.81. Assume that the populations of costs per unit are normally distributed for each type of fuel, and assume that the variances of these populations are equal. Can we conclude, at the 0.10 level of significance, that μ₁, the mean cost per unit for plants using electricity, differs from μ₂, the mean cost per unit for plants using gas? Perform a two-tailed test. Then complete the parts below. Carry your intermediate computations to three or more decimal places and round your answers as specified in the table. (If necessary, consult a list of…
The void volume within a textile fabric affects comfort, flammability, and insulation properties. Permeability of a fabric refers to the accessibility of void space to the flow of a gas or liquid. An article gave summary information on air permeability (cm3/cm2/sec) for a number of different fabric types. Consider the following data on two different types of plain-weave fabric: Fabric Type Sample Size Sample Mean Sample Standard Deviation Cotton 10 51.41 0.75 Triacetate 10 132.15 3.59 Assuming that the porosity distributions for both types of fabric are normal, let's calculate a confidence interval for the difference between true average porosity for the cotton fabric and that for the acetate fabric, using a 95% confidence level. Before the appropriate t critical value can be selected, df must be determined: df = 0.5625 10 + 12.8881 10 2 (0.5625/10)2 9 + (12.8881/10)2 9 = 1.8092 0.1849…
Unoccupied seats on flights cause airlines to lose revenue. As an intern in the finance department for Skyline Airways, Rebecca is tasked with estimating the average number of unoccupied seats per flight over the past year. To accomplish this, the records of 231 flights are randomly selected and the number of unoccupied seats is noted for each of the sampled flights. The sample mean is 14.7 seats and the standard deviation is 3.4 seats.Construct a 90% confidence interval for the population mean number of unoccupied seats per flight during the past year.If necessary, round results to 1 decimal places.
An industrial plant wants to determine which of two types of fuel, electric or gas, is more cost efficient (measured in cost per unit of energy). Independent random samples were taken of plants using electricity and plants using gas. These samples consisted of 7 plants using electricity, which had a mean cost per unit of $64.13 and standard deviation of $8.32, and 12 plants using gas, which had a mean of $60.30 and standard deviation of $8.52. Assume that the populations of costs per unit are normally distributed for each type of fuel, and assume that the variances of these populations are equal. Can we conclude, at the 0.10 level of significance, that u,, the mean cost per unit for plants using electricity, differs from u, the mean cost per unit for plants using gas? Perform a two-tailed test. Then complete the parts below. Carry your intermediate computations to three or more decimal places and round your answers as specified in the table. (If necessary, consult a list of formulas.)…
You are a researcher assessing self-checkout lane violations at supermarkets in a town in the southern United States. The supermarket has five (5) self-checkout lanes. The violations by each lane are as follows: Lane A: 5 violations Lane B: 10 violations Lane C: 15 violations Lane D: 20 violations Lane E: 25 violations Please calculate the following (your standard deviation is 7.91): Mean: Standard Error of the Mean: Please interpret your Standard Error of the Mean.

SEE MORE QUESTIONS

Recommended textbooks for you

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

SEE MORE TEXTBOOKS