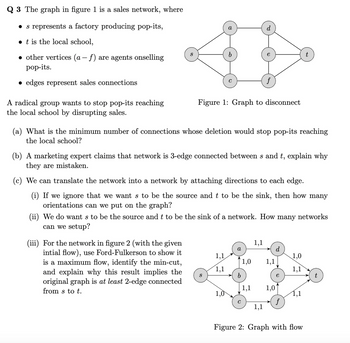
Q 3 The graph in figure 1 is a sales network, where • s represents a factory producing pop-its, ⚫t is the local school, - • other vertices (a – f) are agents onselling pop-its. edges represent sales connections S t A radical group wants to stop pop-its reaching the local school by disrupting sales. f Figure 1: Graph to disconnect (a) What is the minimum number of connections whose deletion would stop pop-its reaching the local school? (b) A marketing expert claims that network is 3-edge connected between s and t, explain why they are mistaken. (c) We can translate the network into a network by attaching directions to each edge. (i) If we ignore that we want s to be the source and t to be the sink, then how many orientations can we put on the graph? (ii) We do wants to be the source and t to be the sink of a network. How many networks can we setup? (iii) For the network in figure 2 (with the given intial flow), use Ford-Fulkerson to show it is a maximum flow, identify the min-cut, and explain why this result implies the original graph is at least 2-edge connected from s to t. 1,1 a d 1,1 1,0 1,0 1,1 1,1 1,1 S b t 1,1 1,0 1,0 с f 1,1 Figure 2: Graph with flow

A:

A: Given,                 1 m = 3.3 ft

A: The normal distribution: A continuous random variable X is said to follow normal distribution if the…

A:

A:

A:

A:

A: Detailed explanation:In the stable matching hassle involving 4 groups—Anthropic, Google, Meta, and…

A:

A: Area of newspaper can be calculated knowing the dimension of the newspaper

A: i. Find an augmenting path from s (source) to t (sink) in the graph first by using Breadth First…

A:

A: As per our guidelinse we can answer only 3 subparts of the given equation. So kindly repost the…

A:

A:

A: The formula to compute the given value is,

A: Let X be the time students study per week and Y be their final exam scores. Given: Sample size (n) =…