
Elementary Geometry For College Students, 7e
7th Edition
ISBN: 9781337614085
Author: Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher: Cengage,
expand_more
expand_more
format_list_bulleted
Question
Calculate the length of each side (round tenths):
![**Algebraic Justification and Distance Calculation**
1. **Prove your justification algebraically**
2. **Calculate the length of each side (round to the nearest tenth):**
\[
\begin{array}{|c|c|}
\hline
d_{AB} & d_{AD} \\
\hline
d_{DC} & d_{BC} \\
\hline
\end{array}
\]
- The first cell calculates the distance \( d_{AB} \) (side AB).
- The second cell calculates the distance \( d_{AD} \) (side AD).
- The third cell calculates the distance \( d_{DC} \) (side DC).
- The fourth cell calculates the distance \( d_{BC} \) (side BC).
Calculations involve finding the distances between the points on a coordinate plane using the distance formula:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
Each resulting distance should be rounded to the nearest tenth of a unit.](https://content.bartleby.com/qna-images/question/b0f86f55-e815-41ae-9a8a-b95a46610cfb/c2103cdf-4ee6-4511-99f9-83945bc1d02b/j0683nr_thumbnail.png)
Transcribed Image Text:**Algebraic Justification and Distance Calculation**
1. **Prove your justification algebraically**
2. **Calculate the length of each side (round to the nearest tenth):**
\[
\begin{array}{|c|c|}
\hline
d_{AB} & d_{AD} \\
\hline
d_{DC} & d_{BC} \\
\hline
\end{array}
\]
- The first cell calculates the distance \( d_{AB} \) (side AB).
- The second cell calculates the distance \( d_{AD} \) (side AD).
- The third cell calculates the distance \( d_{DC} \) (side DC).
- The fourth cell calculates the distance \( d_{BC} \) (side BC).
Calculations involve finding the distances between the points on a coordinate plane using the distance formula:
\[ d = \sqrt{(x_2 - x_1)^2 + (y_2 - y_1)^2} \]
Each resulting distance should be rounded to the nearest tenth of a unit.
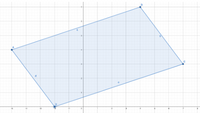
Transcribed Image Text:### Understanding Graph Coordinates: Parallelogram on the Cartesian Plane
#### Description:
The image presents a parallelogram plotted on a Cartesian coordinate system. The graph uses a grid to help visualize the points and lines making up the shape. It illustrates a geometric concept using a coordinate system to define the precise location of each vertex of the parallelogram.
#### Detailed Explanation:
The parallelogram is defined by four vertices A, B, C, and D. These vertices are connected sequentially to form the four sides of the parallelogram.
- **Vertices Coordinates**:
- Vertex A: Situated at coordinates (-4, 2)
- Vertex B: Situated at coordinates (2, 7)
- Vertex C: Situated at coordinates (6, 2.5)
- Vertex D: Situated at coordinates (0, -3)
- **Connecting Lines**:
- Line segment AB: Connects vertex A and B.
- Line segment BC: Connects vertex B and C.
- Line segment CD: Connects vertex C and D.
- Line segment DA: Connects vertex D and A.
#### Characteristics:
- **Parallelism**:
Each pair of opposite sides (AB, CD and AD, BC) are equal in length and parallel to each other, a defining property of a parallelogram.
- **Axes and Coordinates**:
The x-axis and y-axis intersect at the origin (0,0), which acts as a central reference point. The grid lines mark both positive and negative values on the x and y axes, from -10 to 10, convenient for plotting and referencing coordinates.
- **Shading**:
The inside of the parallelogram is shaded to distinguish the shape clearly from the rest of the grid. This shading helps in visualizing the entire enclosed area formed by the vertices and connecting lines.
This graph effectively demonstrates the geometric principle and provides a precise visual aid for understanding how a parallelogram is formed and defined within a coordinate plane using Cartesian coordinates.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps

Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, geometry and related others by exploring similar questions and additional content below.Similar questions
- Sawyer is 5.8 feet tall and notices his shadow at a certain time of day is 3 feet long. He notices the crossbar in his rugby match has a shadow that is 4 ½ feet long. How high does he have to kick the rugby ball to get it over the actual crossbar? Round to the nearest tenth if necessary.arrow_forwardFind the perimeter of the following shape, rounded to the nearest tenth: -5 -5 4 10 11.3 12 14.1arrow_forward10 inches is cut from a board. The remaining length is 85 and 3/16 inches. The saw kerf is 3/16 inch. What was the original length of the board?arrow_forward
- Find the area of the following circle: 21 ft O 346.36 365 17 O321.54 315 22arrow_forwardJun made a round tablecloth for his kitchen table. The tablecloth is 4.5 feet in diameter. Approximately how much fabric did Jun use for the tablecloth? 14 ft.2 32 ft.? 64 ft.? 16 ft.?arrow_forwardA 10" pie was brought to a party. At the end of the party, 3 of it was gone. How 4 many square inches of pie were eaten? 235 4in 2arrow_forward
- How many yards of lace trim is needed to make borders for two rectangular tablecloths each with width 4 ft. and length 6 ft.?arrow_forwardCoach got room is 27ft. by 25ft. Height of this room is 15ft.A volleyball is 31.2inches in diameter. How many volleyballs are needed to fill the roomarrow_forwardCindy ran a race that was 5 kilometers long. W hat was the length of the race in meters?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage,
Elementary Geometry For College Students, 7eGeometryISBN:9781337614085Author:Alexander, Daniel C.; Koeberlein, Geralyn M.Publisher:Cengage, Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning
Elementary Geometry for College StudentsGeometryISBN:9781285195698Author:Daniel C. Alexander, Geralyn M. KoeberleinPublisher:Cengage Learning

Elementary Geometry For College Students, 7e
Geometry
ISBN:9781337614085
Author:Alexander, Daniel C.; Koeberlein, Geralyn M.
Publisher:Cengage,

Elementary Geometry for College Students
Geometry
ISBN:9781285195698
Author:Daniel C. Alexander, Geralyn M. Koeberlein
Publisher:Cengage Learning