Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
Prove that {x_n} converges. Need to use info in a) and b)
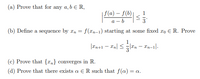
Transcribed Image Text:(a) Prove that for any a, b e R,
| f(a) – f(b)|
а - b
(b) Define a sequence by rn = f(xn-1) starting at some fixed ro E R. Prove
|In+1 – In| <|Tn – Tn-1|-
(c) Prove that {Tn} converges in R.
(d) Prove that there exists a E R such that f(a) = a.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps
Knowledge Booster
Learn more about
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, advanced-math and related others by exploring similar questions and additional content below.Similar questions
- Pennstase 2) 2Determine whether the given sequence converges or n2+ 1 ldiverges. If it converges, calculate its limit: an=(-2)" A/converyes to o B)seauence diveges c)converges to D/converges to 1 3n²+10 Converges toarrow_forward7) The sequence an = In (n+1) In (2n+1) a) Converges to 2 b) Converges to 1 c) Diverges d) None of the abovearrow_forwardn! diverges because an > 0 for alln > 1. True False Docot Coloctionarrow_forward
- 3) ) Use the First Derivative Test to prove that the sequence a, = tanh nis monotonic and bounded for n > 1. (Hint: To prove boundedness, one thing you must do is calculate lim an.)arrow_forward29) Identifa of convergen'ce af a power seri's Ž (-3) nx the itervel n-l Ansus: a ) b) c) d) -32x23 e) 3arrow_forwardWhat theorem sometimes enables us to use l’Hôpital’s Rule to calculate the limit of a sequence? Give an example.arrow_forward
- let {f} bacauchy sequence in anormed. Space X-Show that there exists as bsequence such that UKEN 11 fukt-fnell <2²arrow_forwardA) ~ V2nn en SELECT ALL APPLICABL -1 by LCT B) diverges by ratio test C) diverges D) ~ by RatioTest E) converges by Ratio Test en F) converges G) diverges by root test H) - by Limit Comparison Test en n-1arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,