
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
General Tensor
(3.22) definition to solve in the other image
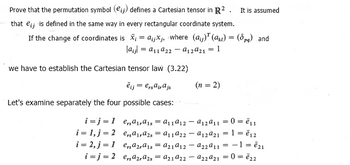
Transcribed Image Text:Prove that the permutation symbol (e) defines a Cartesian tensor in R². It is assumed
that e; is defined in the same way in every rectangular coordinate system.
If the change of coordinates is x₁ = a₁x₁, where (a) (a) = (Spg) and
|a₁|= 9₁1 922 912921 = 1
we have to establish the Cartesian tensor law (3.22)
ēj=ersair a js
Let's examine separately the four possible cases:
i=j=1
i=1, j = 2
i=2, j = 1
i=j=2
(n = 2)
e9₁a1s = a11912
912911 = 0 =ē11
e,,a1ra28 = a₁1922
912921 = 1 = 12
e,a2ra 18 =a21a12-a22911 = -1 = ²21
ersa₂, a28 = a21922-a22921 = 0 = ²22
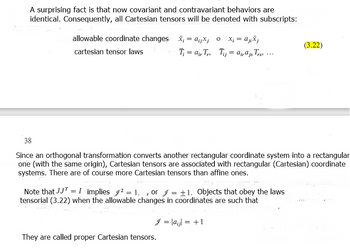
Transcribed Image Text:A surprising fact is that now covariant and contravariant behaviors are
identical. Consequently, all Cartesian tensors will be denoted with subscripts:
allowable coordinate changes ;=A₁jXj ○ x₁ = AjjXj
cartesian tensor laws
T₁ = air Tr Tij = air a js Trs, ...
38
Since an orthogonal transformation converts another rectangular coordinate system into a rectangular
one (with the same origin), Cartesian tensors are associated with rectangular (Cartesian) coordinate
systems. There are of course more Cartesian tensors than affine ones.
Note that JJT = I implies ² = 1₁, or = +1. Objects that obey the laws
tensorial (3.22) when the allowable changes in coordinates are such that
J = |a₁|= +1
(3.22)
They are called proper Cartesian tensors.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 18 images

Knowledge Booster
Similar questions
- Perform indicated operations (4-3i)(2-i)-(3-i)(3+i) How do I go about solving this? How do I distribute the negative sign properly?arrow_forwardForce F1=100 N has an angle of 30° with positive x-axis and Force F2=200 N has an angle of -60° with positive x-axis. Match the values for F1x, F1y, F2x,F2y, Sum of Fx, Sum of Fy, and FR.arrow_forwardTwo forces of magnitudev50N and 20N act on an object at 30 and -45 degrees with the positive x axis. Find F1+F2arrow_forward
- Let u = 6i +6j, v = 3i - j, and w= -7i. Find 5u - (2v - w). 5u - (2v-w) = (Type your answer in terms of i and j.)arrow_forwardPlotthe inverse 11'5-) (-3,-3) (4,-6)arrow_forwardLet A- (a, a2 a3} and B= (b, bz b; be bases for a vector space V, and suppose b, = 2a, - 4az, b,= -a, + 4az, by =a, +az + 3a,. %3D %3D a Find the change of-coordinates matrix from B to A b. Find (x, for x=b, - 2b, + 2b,arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

