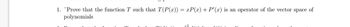
"Prove that the function T such that T(P(x)) = = = xP(x) + P'(x) is an operator of the vector space of polynomials

Let Pn denote the vector space of polynomials in the variable x of degree ʼn or less with real coefficients. Let D : P3 → P₂ be the function that sends a polynomial to its derivative. That is, D(p(x)) = p′ (x) for all polynomials p(x) = P3. Is D a linear transformation? Let p(x) = a3x³ + ª²x² + ª₁x + ªº and q(x) = b3x³ + b₂x² + b₁x + bo be any two polynomials in P3 and c € R. a. D(p(x) + q(x)) : = D(p(x)) + D(q(x)) : = + Does D(p(x) + q(x)) = D(p(x)) + D(q(x)) for all p(x), q(x) = P3? choose b. D(cp(æ)) = c(D(p(x))) = Does D(cp(x)) = c(D(p(x))) for all c € R and all p(x) = P3? choose c. Is D a linear transformation? choose (Enter a3 as a3, etc.)

Let denote the vector space of polynomials in the variable x of degree n or less with real coefficients. Let D: 03 → be the function that sends a polynomial to its derivative. That is, D(p(x)) = p'(x) for all polynomials p(x) E 3. Is D a linear transformation? Let p(x) = a3x³ + a₂x² + a₁x + aº and q(x) = b3x³ + b₂x² + b₁x + bo be any two polynomials in 3 and c E R. a. D(p(x) + q(x)) = D(p(x)) + D(q(x)) = Does D(p(x) + q(x)) = D(p(x)) + D(q(x)) for all p(x), q(x) = ? choose b. D(cp(x)) = c(D(p(x))) = Does D(cp(x)) = c(D(p(x))) for all c ER and all p(x) E 3? choose c. Is D a linear transformation? choose . (Enter a3 as a3, etc.)

1.T.2 Let V be the set of all twice-differentiable functions f : R → R satisfying f"(x) = f(x) – 5. Is V a vector space? Why or why not?

, Let C" (R) be the vector space of "smooth" functions, i.e., real-valued functions f(x) in the variable z that have infinitely many derivatives at all points x E R. Let D: C* (IR) → C¤(R) and D² : C∞ (R) → C°(R) be the linear transformations defined by the first derivative D(f(x)) = f'(x) and the second derivative D²(f(x)) = f"(x). a. Determine whether the smooth function g(x) = 7e1z is an eigenvector of D. If so, give the associated eigenvalue. If not, enter NONE. Eigenvalue = b. Determine whether the smooth function h(x) = sin(9x) is an eigenvector of D2. If so, give the associated eigenvalue. If not, enter %3D NONE. Eigenvalue =

Horizontal cross-sections of the vector fields F⃗ (x,y,z) and G⃗ (x,y,z) are given in the figure. Each vector field has zero z-component (i.e., all of its vectors are horizontal) and is independent of z (i.e., is the same in every horizontal plane). You may assume that the graphs of these vector fields use the same scale. (a) Are div(F⃗ ) and div(G⃗ ) positive, negative, or zero at the origin? Be sure you can explain your answer. At the origin, div(F⃗ ) is At the origin, div(G⃗ ) is (b) Are F⃗ and G⃗ curl free (irrotational) or not at the origin? Be sure you can explain your answer. At the origin, F⃗ is At the origin, G⃗ is (c) Is there a closed surface around the origin such that F⃗ has nonzero flux through it? Be sure you can explain your answer by finding an example or a counterexample. (d) Is there a closed surface around the origin such that G⃗ has nonzero circulation around it? Be sure you can explain your answer by finding an example or a…

Horizontal cross-sections of the vector fields F⃗ (x,y,z) and G⃗ (x,y,z) are given in the figure. Each vector field has zero z-component (i.e., all of its vectors are horizontal) and is independent of z (i.e., is the same in every horizontal plane). You may assume that the graphs of these vector fields use the same scale. (a) Are div(F⃗ ) and div(G⃗ ) positive, negative, or zero at the origin? Be sure you can explain your answer. At the origin, div(F⃗ ) is Choose At the origin, div(G⃗ ) is Choose (b) Are F⃗ and G⃗ curl free (irrotational) or not at the origin? Be sure you can explain your answer. At the origin, F⃗ is Choose At the origin, G⃗ is

The linear operator L : R² R² given by L (²) 6x - a) (55x + y) = -1 Which of the following gives a formula for L-¹(x) ? b) (x + y) 6x x + y 5x + 6y, is a vector space isomorphism. c) (5x-by) d) (x - 5y) = e) none

Linear Algebra

Let q = - 2xy - y² + 2xz+2yz + z² be a quadratic form on R³ viewed as a polynomial in 3 variables. Find a linear change of variables tou, v, w that puts q into the canonical form in `Sylvester's law of inertia'!. What are the values of the associated indices s, t? Select one: O We let u = √3(x − y + 2), v = x+y, w = (x - y)/2 to find that q = u²+². Hence s = 2, t = 0 in Sylvester's law of intertia. O We let u = x - 2y, v = z+y, w = √3y to find that q=u²v² w². Hence s = 1,t = 2 in Sylvester's law of intertia. O we let u = x, v= √2(x+y), w = x+y+z to find that q = u²v² + w²2. Hence s = 2, t = 1 in Sylvester's law of intertia. O None of the others apply O The quadratic form does not obey the condition to be diagonalisable over R. This is because the minimal polynomial of the corresponding matrix is not a product of distinct linear factors. Hence Sylvester's law of inertia does not apply. By convention, we set s = t = ∞ when this happens.