Question
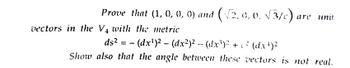
Transcribed Image Text:Prove that (1, 0, 0, 0) and (√2,0,0. √3/c) are unit
vectors in the V₁ with the metric
ds²
· (dx¹)² – (dx²)² -- (dx³)² + c² (dx¹)²
Show also that the angle between these vectors is not real.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- Given vectors a=(5,3) and b=(-1,-2) Find the x-component of the resultant vector: T` = 2 a + 36arrow_forwardUsing spherical polar coordinates r, 0, p to find CM of a uniform solid hemisphere of radius R, whose flat face lies in the xy plane with its center at the origin. The element of volume is in spherical polars of dV = r² dr sine de dip.arrow_forwardEvaluate the integral. dx (x – 4)(x – 3)(x + 5) | (Use symbolic notation and fractions where needed. Use C for the arbitrary constant.) dx %3D J (x – 4)(x – 3)(x + 5)arrow_forward
arrow_back_ios
arrow_forward_ios