Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Related questions
Question
Parts 65-68 please. Use second image as reference
![Problem 6. Find a linear polynomial which is the best least
squares fit to the following data:
We are looking for a function f(x) = C₁ + C₂x, where C₁, C₂
are unknown coefficients. The data of the problem give rise
to an overdetermined system of linear equations in variables c₁
and C₂:
A =
This system is inconsistent.
X -2 -1 0 1 2
f(x) -3 -2 1 2 5
We can represent the system as a matrix equation Ac = y,
where
1
-2 -1 0
1 1 1
1
1
1
1
1
1
1
2
-2
-1
0
1
2
C₁2C₂ = -3,
C₁-C₂ = -2,
C₁ = 1,
C₁ + C₂ = 2,
C₁ + 2C₂ = 5.
The least squares solution c of the above system is a solution
of the normal system AT Ac = ATy:
1
1 -2
1
C =
1 1 1 1 1
(0-44])
-2 -1 0 1 2
1 1
C1
C₂
-3
-2
-0
y =
1
2
5
1 2
(5) (2) (3)
=
C₁ = 3/5
C₂ = 2
-3
-2
1
2
Thus the function f(x) = 3 + 2x is the best least squares fit
to the above data among linear polynomials.](https://content.bartleby.com/qna-images/question/8b19493d-2e0b-4edb-977e-29215d3bb0dd/f6d481f2-d9b9-4bfd-95e2-89f324ff4d28/3cy480k_processed.jpeg)
Transcribed Image Text:Problem 6. Find a linear polynomial which is the best least
squares fit to the following data:
We are looking for a function f(x) = C₁ + C₂x, where C₁, C₂
are unknown coefficients. The data of the problem give rise
to an overdetermined system of linear equations in variables c₁
and C₂:
A =
This system is inconsistent.
X -2 -1 0 1 2
f(x) -3 -2 1 2 5
We can represent the system as a matrix equation Ac = y,
where
1
-2 -1 0
1 1 1
1
1
1
1
1
1
1
2
-2
-1
0
1
2
C₁2C₂ = -3,
C₁-C₂ = -2,
C₁ = 1,
C₁ + C₂ = 2,
C₁ + 2C₂ = 5.
The least squares solution c of the above system is a solution
of the normal system AT Ac = ATy:
1
1 -2
1
C =
1 1 1 1 1
(0-44])
-2 -1 0 1 2
1 1
C1
C₂
-3
-2
-0
y =
1
2
5
1 2
(5) (2) (3)
=
C₁ = 3/5
C₂ = 2
-3
-2
1
2
Thus the function f(x) = 3 + 2x is the best least squares fit
to the above data among linear polynomials.
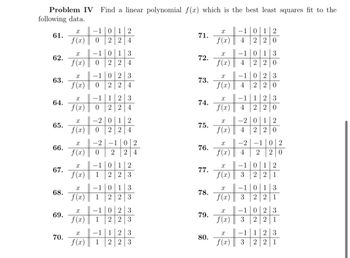
Transcribed Image Text:Problem IV Find a linear polynomial f(x) which is the best least squares fit to the
following data.
X -1 0 1 2
f(x)
0 224
61.
62.
63.
64.
65.
66.
67.
68.
69.
70.
X -1 0 1 3
224
f(x) 0
X -1
H
f(x)
X
f(x)
X
f(x)
X
f(x)
X
f(x)
X
f(x)
X
f(x)
X
f(x)
0
023
22 4
-1
1 2 3
0 224
-201 2
0 22 4
-2 -1 02
0 2 24
-1 012
1 223
-1 0 1 3
1 223
-1 023
1 22 3
-1 1 2 3
1 2 2 3
71.
72.
73.
74.
75.
76.
77.
78.
79.
80.
X -1 012
f(x) 4 220
X -1 013
4 220
f(x)
X
f(x)
Xx
f(x)
X
f(x)
X
f(x)
X
f(x)
-1 023
4 220
X
-2 -1 02
f(x) 4 2 20
X
f(x)
-1
1 2 3
4 2 20
-2 012
4
20
-1 0 1 2
3 22 1
-1 0 1 3
3 22 1
-1 023
3 22 1
X
-1
f(x) 3
1 23
21
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 6 steps
Knowledge Booster
Similar questions
- Unit 1 Section B Free Response. 1. Find the width and height of an older 50-inch television whose screen has an aspect ratio oarrow_forwardEquation: Answer: -29802 RM 4. A city of 100,000 is having pollution problems and is decreasing in size 2% annually (every year). Find the population of this city in 100 years. Equation: Answer: 5. In 1982, the number of Starbucks was 5 shops. It has exponentially grown by 21% yearly. Let t= the number of years since 1982. Find an equation for this growth and find the number of Starbucks predicted in 2015. Equation: Answer: 6. A computer's value declines about 7% yearly. Sally bought a computer for $800 in 2005. How much is it worth in 2009.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
- Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY 
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,