3 K 5 8 K -4.2 -2.5 0 1.7 d(1.7, 2.5) 11.7 (-2.5) |= 4.2 - = - d(3, 8) 13 81-5 Fig. 2. Distance on R x, y = R. Figure 2 illustrates the notation. In the plane and in “ordi- nary" three-dimensional space the situation is similar. In functional analysis we shall study more general "spaces" and "functions" defined on them. We arrive at a sufficiently general and flexible concept of a "space" as follows. We replace the set of real numbers underlying R by an abstract set X (set of elements whose nature is left unspecified) and introduce on X a "distance function" which has only a few of the most fundamental properties of the distance function on R. But what do we mean by "most fundamental"? This question is far from being trivial. In fact, the choice and formula- tion of axioms in a definition always needs experience, familiarity with practical problems and a clear idea of the goal to be reached. In the present case, a development of over sixty years has led to the following concept which is basic and very useful in functional analysis and its applications. 1.1-1 Definition (Metric space, metric). A metric space is a pair (X, d), where X is a set and d is a metric on X (or distance function on X), that is, a function defined² on XXX such that for all x, y, z= X we have: (M1) d is real-valued, finite and nonnegative. (M2) d(x, y)=0 if and only if x = y. (M3) d(x, y) = d(y, x) (Symmetry). (M4) d(x, y)d(x, z)+d(z, y) (Triangle inequality). 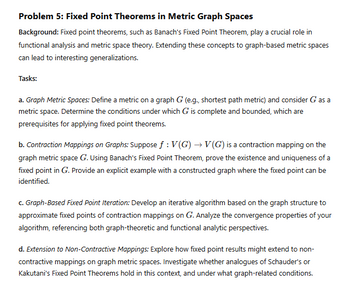■ Problem 5: Fixed Point Theorems in Metric Graph Spaces Background: Fixed point theorems, such as Banach's Fixed Point Theorem, play a crucial role in functional analysis and metric space theory. Extending these concepts to graph-based metric spaces can lead to interesting generalizations. Tasks: a. Graph Metric Spaces: Define a metric on a graph G (e.g., shortest path metric) and consider G as a metric space. Determine the conditions under which G is complete and bounded, which are prerequisites for applying fixed point theorems. b. Contraction Mappings on Graphs: Suppose ƒ : V(G) → V(G) is a contraction mapping on the graph metric space G. Using Banach's Fixed Point Theorem, prove the existence and uniqueness of a fixed point in G. Provide an explicit example with a constructed graph where the fixed point can be identified. c. Graph-Based Fixed Point Iteration: Develop an iterative algorithm based on the graph structure to approximate fixed points of contraction mappings on G. Analyze the convergence properties of your algorithm, referencing both graph-theoretic and functional analytic perspectives. d. Extension to Non-Contractive Mappings: Explore how fixed point results might extend to non- contractive mappings on graph metric spaces. Investigate whether analogues of Schauder's or Kakutani's Fixed Point Theorems hold in this context, and under what graph-related conditions.

In part a, can you please help me describe the range and the kernel of T in geometric terms like if it's a line, plane,... ?

4. Give a geometric description of each family of planes.

Many smartphones are now able to use information sent out by GPS satellites (of which there are about nine overhead at any one time) in a similar way. Since the satellites are moving around in space, and therefore can’t be located on a two-dimensional map of the Earth, we now need to consider three-dimensional geometry. How many satellites are needed to be sure of the location of a smartphone? Explain your findings

The minimum vertical dimension (B) for the junction box shown in the Figure is _______

Part A: What are the coordinates of point A if you move it to the second quadrant tocreate isosceles triangle CAT?

Section L.2 Chapter 1 16. A manufacturer must describe a dimensioned part according to its x & y coordinates for a CNC (computer numerically controlled) od I G machine to produce it. These coordinates are る 5 measured relative to a datum. The x- coordinate is the horizontal distance from the datum, right is positive and left is negative The y-coordinate is the vertical distance from the datum, up is positive and down is negative. Fill in the chart that locates the holes and points that define the part. I G DATUM 4 2 P4 X - Coordinate Y - Coordinate P1 P3 13 7 PIO P5 P7 H I Pe P8 P10 H1 H2 H4 H5 H7 38

A specific point (0,b) is known as the __________