Question
thumb_up100%
can you please answer (e) & (f) & (g)?
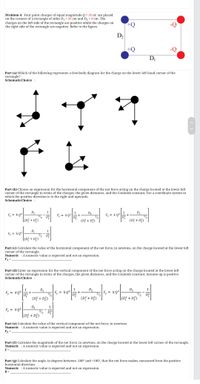
Transcribed Image Text:Problem 4: Four point charges of equal magnitude Q = 35 nC are placed
on the corners of a rectangle of sides D1 = 26 cm and D, = 8 cm. The
charges on the left side of the rectangle are positive while the charges on
the right side of the rectangle are negative. Refer to the figure.
D2
+Q
DI
Part (a) Which of the following represents a free-body diagram for the charge on the lower left hand corner of the
rectangle?
SchematicChoice :
Part (b) Choose an expression for the horizontal component of the net force acting on the charge located at the lower left
corner of the rectangle in terms of the charges, the given distances, and the Coulomb constant. Use a coordinate system in
which the positive direction is to the right and upwards.
SchematicChoice :
D2
3/2 DE
[(D} + D3)
1
D2
3/2
(D; + D3)
1
F = k Q?
F, = kQ?
+
F = k Q2
(Dž + D;)
1
F = kQ²
3/2 D
[(D} + D3)
Part (c) Calculate the value of the horizontal component of the net force, in newtons, on the charge located at the lower left
corner of the rectangle.
Numeric : A numeric value is expected and not an expression.
Fx =
Part (d) Enter an expression for the vertical component of the net force acting on the charge located at the lower left
corner of the rectangle in terms of the charges, the given distances, and the Coulomb constant. Assume up is positive.
SchematicChoice :
D2
3/2
(D? + D?)
D2
3/2
[(D} + D?)
1
D1
F, = kQ²
3/2
Fy = kQ2
F, = kQ²
(D? + D3)
D1
3/2 D?
[(D} + D3)
1
F, = kQ?
Part (e) Calculate the value of the vertical component of the net force, in newtons.
Numeric : A numeric value is expected and not an expression.
Fy
Part (f) Calculate the magnitude of the net force, in newtons, on the charge located at the lower left corner of the rectangle.
Numeric : Anumeric value is expected and not an expression.
F =
Part (g) Calculate the angle, in degrees between -180° and +180°, that the net force makes, measured from the positive
horizontal direction.
Numeric : A numeric value is expected and not an expression.
Expert Solution
arrow_forward
Step 1

Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios