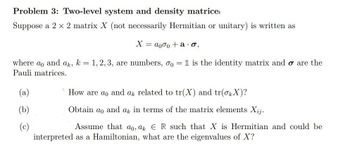
Problem 3: Two-level system and density matrice Suppose a 2 x 2 matrix X (not necessarily Hermitian or unitary) is written as X = aooo + a.o, where ao and ak, k = 1, 2, 3, are numbers, 0 = 1 is the identity matrix and o are the Pauli matrices. (a) (b) How are ao and a related to tr(X) and tr(okX)? Obtain ao and a in terms of the matrix elements Xij.

please answer c) only 2. a) A spinless particle, mass m, is confined to a two-dimensional box of length L. The stationary Schrödinger equation is - +a) v(x, y) = Ev(x, y), for 0 < r, y < L. The bound- ary conditions on ý are that it vanishes at the edges of the box. Verify that solutions are given by 2 v(1, y) sin L where n., ny = 1,2..., and find the corresponding energy. Let L and m be such that h'n?/(2mL²) = 1 eV. How many states of the system have energies between 9 eV and 24 eV? b) We now consider a macroscopic box (L of order cm) so that h'n?/(2mL?) ~ 10-20 eV. If we define the wave vector k as ("", ""), show that the density of states g(k), defined such that the number of states with |k| between k and k +dk is given by g(k)dk, is Ak 9(k) = 27 c) Use the expression for g(k) to show that at room temperature the partition function for the translational energy of a particle in a macroscopic 2-dimensional box is Z1 = Aoq, where 2/3 oq = ng = mk„T/2nh?. Hence show that the average…

a2 Laplacian operator 72 = ax? ay? T əz2 in spherical polar coordinates is given by az? p² = () 1 a 1 1 a2 r2 sin e ae sin 0-) is an eigenfunction of the Laplacian operator and find the +- r2 sin 0 a0 r2 ar ar. r2 sin? 0 a20 sin 0 sin o Show that function r2 corresponding eigenvalue.

The Hamiltonian for the one dimensional quantum oscillator is 1 p² 1 Ĥ = 1² + ½ k²² = 12 + √ mw² ಠ2m 2m 2 where k = mw². 1) Define the operators ₁₁ and ₁₁ such that Ĥ = ½ħw (p² + ²). Define Ĥ2 as a function of 1 and p₁ such that Ĥ = hwĤ₂. - 2) Let us define the new operators â (1 + i₁) and ↠= ½(î₁ — ip₁). Express ₁ and p₁ as a function of â and â³. Knowing that [^^1,î₁] = i and [1, 1] = -i, calculate âât and â†â. Express Ĥ2 as a function of a and at. 3) Let us define Ñ such that Ĥ₂ = Ñ + ½. Knowing that Ĥ, Ĥ₂ and Ñ have the same eigenstates, what are their corresponding eigenvalues?