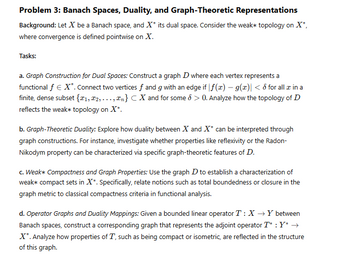
Problem 3: Banach Spaces, Duality, and Graph-Theoretic Representations Background: Let X be a Banach space, and X* its dual space. Consider the weak* topology on X*, where convergence is defined pointwise on X. Tasks: a. Graph Construction for Dual Spaces: Construct a graph D where each vertex represents a functional ƒ € X*. Connect two vertices ƒ and g with an edge if |f(x) - g(x)| < 6 for all x in a finite, dense subset {1, 2,..., n} CX and for some 6 > 0. Analyze how the topology of D reflects the weak* topology on X*. b. Graph-Theoretic Duality: Explore how duality between X and X* can be interpreted through graph constructions. For instance, investigate whether properties like reflexivity or the Radon- Nikodym property can be characterized via specific graph-theoretic features of D. c. Weak* Compactness and Graph Properties: Use the graph D to establish a characterization of weak* compact sets in X*. Specifically, relate notions such as total boundedness or closure in the graph metric to classical compactness criteria in functional analysis. d. Operator Graphs and Duality Mappings: Given a bounded linear operator T: XY between Banach spaces, construct a corresponding graph that represents the adjoint operator T* : Y* → X*. Analyze how properties of T, such as being compact or isometric, are reflected in the structure of this graph. 3 K 5 8 K -4.2 -2.5 0 1.7 d(1.7, 2.5) 11.7 (-2.5) |= 4.2 - = - d(3, 8) 13 81-5 Fig. 2. Distance on R x, y = R. Figure 2 illustrates the notation. In the plane and in “ordi- nary" three-dimensional space the situation is similar. In functional analysis we shall study more general "spaces" and "functions" defined on them. We arrive at a sufficiently general and flexible concept of a "space" as follows. We replace the set of real numbers underlying R by an abstract set X (set of elements whose nature is left unspecified) and introduce on X a "distance function" which has only a few of the most fundamental properties of the distance function on R. But what do we mean by "most fundamental"? This question is far from being trivial. In fact, the choice and formula- tion of axioms in a definition always needs experience, familiarity with practical problems and a clear idea of the goal to be reached. In the present case, a development of over sixty years has led to the following concept which is basic and very useful in functional analysis and its applications. 1.1-1 Definition (Metric space, metric). A metric space is a pair (X, d), where X is a set and d is a metric on X (or distance function on X), that is, a function defined² on XXX such that for all x, y, z= X we have: d is real-valued, finite and nonnegative. (M1) (M2) d(x, y)=0 if and only if x = y. (M3) d(x, y) = d(y, x) (Symmetry). (M4) d(x, y)d(x, z)+d(z, y) (Triangle inequality). ■

Let max(a, b) denote the maximum and min(a, b) denote the minimum of the real numbers a and b. For example, max(2, 5) = 5 and min(-1, -2) = -2. [Hint: Take cases and write these expressions without absolute values.] (a) Prove that max(a, b) = 3 + b + |a - bl. +b+ Suppose that a > b, so max(a, b) = a and la - b| = a - b. Then +b+ la - b On the other hand, if b> a, then max(a, b) = b and la - b| =b -a. In this case, +b+ la - b. a+b+ 2 If a = b, then la - b| - and the result is trivial. (b) Prove that min(a, b) = 3 + b – |a – b|, 2 If a < b, then min(a, b) = a and la - b| = · In this case 3 + b – |a – bl a + b -( 2 Similarly, if b< a, then +b- la – bl - ; and if a = b, the result is trivial.

What is the domain of the relation x(x-3) ? {(X,y): y = (x+4)(x-7)). {x: x = R, x -4, x 2 7} O {x: xE R, x # -4, x * 7} O {x: x : R, x < -4, x * 7} O (x: x ¢ R, x = -4, x 7}

solve 3.61 in detail please  i posted this question on chegg and received the wrong answer so please don't copy and paste the wrong answer.

Using the function f: (-1,2] → R, ƒ (x) = 4x³ – 3x² – 6x + 2, a. Determine all the stationary points within the domain restriction and the nature of each of these turning points b.-Sketch the graph of the function in the space below, indicating the endpoints, y- intercepts, and the turning points. Show calculations for the start and end point in the space below too. ORMITUniversity Page 4 of 7 MM Quiz (2021)

Write a function that returns true (1) if u and v have an edge in a directed graph represented by adjacency lists and false (0) otherwise.

3) In the table below, give the approximate x-coordinate of each discontinuity of the nifty function below AND give the discontinuity type (removable, jump, or infinite). The dashed lines are one unit apart. From smallest to biggest (there may be more rows than necessary): Discontinuity at Туре

-rsity View Academy | x C =uview.instructure.com/courses/10456/quizzes/268436 Distance (meters) Use the graph of m (t) and z (t) to determine all the true statements. 48 44 40 36 32 28 24 20 16 12 8 Algebra 14.9 Comparing Gr 41 0 4 -2(1) 8 12 (m(1) z (20) = m (20) 16 20 24 28 Time (sec.) Live Sessions in() has a greater maximum than the maximum of (!) z(t) and in (t) have the same minimum at (6, 10). in (t) and 2 (1) are both increasing from 22 to 28 seconds. m

Use calculus to find the absolute maximum value and the absolute minimum value, if any, of the function. (If an answer does not exist, enter DNE.) f(x) = -x2 + 4x + 10 [4, 7] on Part 1 of 7 Notice that the domain off is (Enter your answer using interval notation.) and ---Select--- a closed interval. Also, the function f --Select--- a continuous function. Submit Skip (you cannot come back).

Given a function Ax) and g(x) - 3(x-1). how would the graph of y-Ax) compare to the graph of y- Ag(x))? vertically stretched and translated to the right horizontally stretched and translated to the right horizontally compressed and translated to the right horizontally compressed and translated to the left acer