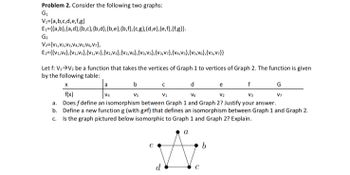
Problem 2. Consider the following two graphs: G₁ V₁=(a,b,c,d,e,f,g) E₁={{a,b),(a,d),(b,c}), (b,d), (b,e},{b,f),{c,g),(d,e},{e,f},{f,g}}. G₂ V2=(V1, V2, V3, V4, VS, V6, V7), E2={{V1,V4},{V₁,VS),{V1,V),(v2,v3), (V2,V6), (V3,VS), (Va,V7},{V4,Vs),(vs,V6},{VS,V7}} Let f: V₁ V₂ be a function that takes the vertices of Graph 1 to vertices of Graph 2. The function is given by the following table: X d f f(x) V4 V6 V₂ V3 a. Does f define an isomorphism between Graph 1 and Graph 2? Justify your answer. b. Define a new function g (with gef) that defines an isomorphism between Graph 1 and Graph 2. c. Is the graph pictured below isomorphic to Graph 1 and Graph 2? Explain. a a b Vs d с V₁ b G V7

Graphing from equations

What is the vertex, focus, and directrix in the graph?

#9

Graph 1 8- 6. 4- 2. Graph 2 Graph 3 -2- -6- • One-to-one? O Yes No O Yes No O Yes No Graph 4. Graph 5. Graph 6 4+ -8-64 -8- -6+ -8- -8-6-4 One-to-one? O Yes No O Yes No O Yes No

Which of the following graphs represents the equation (X-3)²,0 (y-2)2² -1? 9 4 A -6 -6 -5 -- -3 + - -3 --2 -1 -2 -1 5 4 3 2 1 0 -1 -2 -6 5 4 2 1 0 -1 -2 -3 - & -6 5 4 Ty 3 2 1 -3 -2 -1 0 -1 N T & -6 1 1 1 2 3 4 5 3 3 4 4 5 5 6 6 6

Determine the range of the following graph: 12 11 10 8 7 4 3 1 -12-1-10 -9 -8 -7 -6 -5 -4 -3 -2 -1, -1 2 3 4 5 6 7 8 9 10 11 12 1 -3 -4 -5 -6 -7 -8 -9 -10 -11 -12