Problem 2: The switch in Fig.2 closes at t = 0s and, after a very long time, the capacitor is fully charged. Find expressions for the total energy supplied by the battery as the capacitor is being charged, total energy dissipated by the resistor as the capacitor is being charged, and the en- ergy stored in the capacitor when it is fully charged. = power FIG. 2: The scheme for Problem 2 a) In an ideal battery, a charge dq gains potential energy Edq as it moves up the 'charge escalator' inside the battery. Therefore, the p supplied by the battery at each moment of time is Phat &=I& (this expression was derived in paragraph 28.3 of the textbook). The current in an RC circuit with a charging capacitor changes with time as I = Ioe-t/t, where Io = &/R and T = RC (this expression was provided at the end of paragraph 28.9 of the textbook). To compute the total energy supplied by the battery as the capacitor is charging, take the integral Ebat = Phatdt. Express the final answer for Ebat in terms of & and C only. R ww b) The power dissipated by the resistor is equal to Pres= I2R (note that it is a function of time in this problem). Compute the total energy dissipated in the resistor by taking the integral Eres = Prest. Express your answer in terms of & and C only. c) When the capacitor is fully charged, the potential difference between its plates is equal to E, and there is no current flowing in the circuit. Express the potential energy stored in the capacitor in terms of E and C. d) Do your results for parts a) to c) show that the energy is conserved? Explain.
Problem 2: The switch in Fig.2 closes at t = 0s and, after a very long time, the capacitor is fully charged. Find expressions for the total energy supplied by the battery as the capacitor is being charged, total energy dissipated by the resistor as the capacitor is being charged, and the en- ergy stored in the capacitor when it is fully charged. = power FIG. 2: The scheme for Problem 2 a) In an ideal battery, a charge dq gains potential energy Edq as it moves up the 'charge escalator' inside the battery. Therefore, the p supplied by the battery at each moment of time is Phat &=I& (this expression was derived in paragraph 28.3 of the textbook). The current in an RC circuit with a charging capacitor changes with time as I = Ioe-t/t, where Io = &/R and T = RC (this expression was provided at the end of paragraph 28.9 of the textbook). To compute the total energy supplied by the battery as the capacitor is charging, take the integral Ebat = Phatdt. Express the final answer for Ebat in terms of & and C only. R ww b) The power dissipated by the resistor is equal to Pres= I2R (note that it is a function of time in this problem). Compute the total energy dissipated in the resistor by taking the integral Eres = Prest. Express your answer in terms of & and C only. c) When the capacitor is fully charged, the potential difference between its plates is equal to E, and there is no current flowing in the circuit. Express the potential energy stored in the capacitor in terms of E and C. d) Do your results for parts a) to c) show that the energy is conserved? Explain.
Hello, I need help with part A and part B and Part C is there any chance you can help me with those problems and also can you label them as well thank you
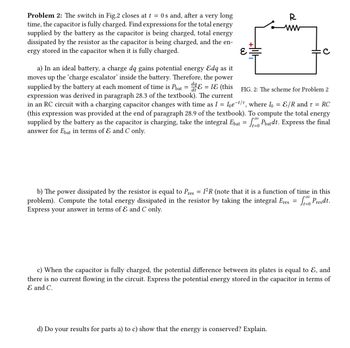
Transcribed Image Text:**Problem 2**: The switch in Fig. 2 closes at \( t = 0 \) s and, after a very long time, the capacitor is fully charged. Find expressions for the total energy supplied by the battery as the capacitor is being charged, total energy dissipated by the resistor as the capacitor is being charged, and the energy stored in the capacitor when it is fully charged.
**Diagram Explanation**:
- The diagram (Fig. 2) shows a simple RC circuit with a battery of EMF \( \mathcal{E} \), a resistor \( R \), and a capacitor \( C \).
**Questions and Explanations**:
**a)** In an ideal battery, a charge \( dq \) gains potential energy \( \mathcal{E} dq \) as it moves up the 'charge escalator' inside the battery. Therefore, the power supplied by the battery at each moment of time is \( P_{\text{bat}} = \frac{dq}{dt} \mathcal{E} = I \mathcal{E} \) (this expression was derived in paragraph 28.3 of the textbook). The current in an RC circuit with a charging capacitor changes with time as \( I = I_0 e^{-t/ \tau} \), where \( I_0 = \frac{\mathcal{E}}{R} \) and \( \tau = RC \) (this expression was provided at the end of paragraph 28.9 of the textbook). To compute the total energy supplied by the battery as the capacitor is charging, take the integral \( E_{\text{bat}} = \int_{t=0}^{\infty} P_{\text{bat}} dt \). Express the final answer for \( E_{\text{bat}} \) in terms of \( \mathcal{E} \) and \( C \) only.
**b)** The power dissipated by the resistor is equal to \( P_{\text{res}} = I^2 R \) (note that it is a function of time in this problem). Compute the total energy dissipated in the resistor by taking the integral \( E_{\text{res}} = \int_{t=0}^{\infty} P_{\text{res}} dt \). Express your answer in terms of \( \mathcal{E} \) and \( C \) only.
**c)** When the capacitor is
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Need a deep-dive on the concept behind this application? Look no further. Learn more about this topic, physics and related others by exploring similar questions and additional content below.
University Physics (14th Edition)PhysicsISBN:9780133969290Author:Hugh D. Young, Roger A. FreedmanPublisher:PEARSON
Introduction To Quantum MechanicsPhysicsISBN:9781107189638Author:Griffiths, David J., Schroeter, Darrell F.Publisher:Cambridge University Press
Physics for Scientists and EngineersPhysicsISBN:9781337553278Author:Raymond A. Serway, John W. JewettPublisher:Cengage Learning
Lecture- Tutorials for Introductory AstronomyPhysicsISBN:9780321820464Author:Edward E. Prather, Tim P. Slater, Jeff P. Adams, Gina BrissendenPublisher:Addison-Wesley
College Physics: A Strategic Approach (4th Editio...PhysicsISBN:9780134609034Author:Randall D. Knight (Professor Emeritus), Brian Jones, Stuart FieldPublisher:PEARSON