
A First Course in Probability (10th Edition)
10th Edition
ISBN: 9780134753119
Author: Sheldon Ross
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
Please do part 3
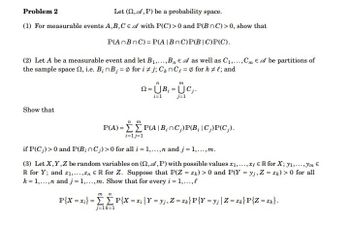
Transcribed Image Text:Problem 2
Let (,,P) be a probability space.
(1) For measurable events A,B,CE A with P(C) >0 and P(BnC)>0, show that
P(AnBnC)=P(A | BnC)P(B|C)P(C).
(2) Let A be a measurable event and let B₁,...,B₁ € A as well as C₁,...,Cm E A be partitions of
the sample space , i.e. B; nB;= for i #j; CnCe = fork #l; and
Show that
n m
P(A)=P(A|B₂nC;)P(B, C;)P(C;).
m n
n
m
Q=ÜB₁ =ÜC₁.
i=1
j=1
i=1j=1
if P(C;) >0 and P(B; nC;) >0 for all i = 1,...,n and j = 1,..., m.
(3) Let X, Y, Z be random variables on (n,A,P) with possible values x₁,...,xe ER for X; y₁,...,ym €
R for Y; and 2₁,...,Zn ER for Z. Suppose that P(Z = zh) >0 and P(Y = yj, Z = zk) > 0 for all
k = 1,...,n and j = 1,...,m. Show that for every i = 1,...,.
j=1k=1
P{X = xi} = [[P{X=xi | Y=yj, Z = zh} P {Y=yj | Z = zk} P {Z = zk}.
Expert Solution
arrow_forward
Step 1
As asked by you, I have answered part 3. Please find the explanation below. Thank you.
Step by stepSolved in 2 steps with 1 images

Knowledge Booster
Similar questions
- A 3rd year student has 75 credits and a cumulative GPA of 2.88. This semester she is registered for 15 credits and hopes to raise his cumulative GPA to 3.00 and make the Dean’s List. Is it possible? What semester GPA will she need to accomplish that? Show your calculations or post a screenshot from your online GPA grade calculator.arrow_forwardignore the question above please just do #7arrow_forwardPlease do #20 as wellarrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 A First Course in Probability (10th Edition)ProbabilityISBN:9780134753119Author:Sheldon RossPublisher:PEARSON
A First Course in Probability (10th Edition)ProbabilityISBN:9780134753119Author:Sheldon RossPublisher:PEARSON

A First Course in Probability (10th Edition)
Probability
ISBN:9780134753119
Author:Sheldon Ross
Publisher:PEARSON
