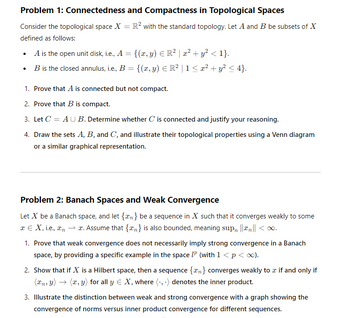
Problem 1: Connectedness and Compactness in Topological Spaces Consider the topological space X = R² with the standard topology. Let A and B be subsets of X defined as follows: • • A is the open unit disk, i.e., A = {(x, y) = R² | x² + y² < 1}. B is the closed annulus, i.e., B = {(x, y) Є R² | 1 ≤ x² + y² ≤ 4}. 1. Prove that A is connected but not compact. 2. Prove that B is compact. 3. Let C AUB. Determine whether C is connected and justify your reasoning. 4. Draw the sets A, B, and C, and illustrate their topological properties using a Venn diagram or a similar graphical representation. Problem 2: Banach Spaces and Weak Convergence Let X be a Banach space, and let {n} be a sequence in X such that it converges weakly to some x = X, i.e., xnx. Assume that {n} is also bounded, meaning sup ||xn|| < ∞. 1. Prove that weak convergence does not necessarily imply strong convergence in a Banach space, by providing a specific example in the space IP (with 1 < p < ∞). 2. Show that if X is a Hilbert space, then a sequence {n} converges weakly to a if and only if (xn, y) (x, y) for all y € X, where (.,.) denotes the inner product. 3. Illustrate the distinction between weak and strong convergence with a graph showing the convergence of norms versus inner product convergence for different sequences.

#11

If V1, V2, V3, V4, and W are all vector spaces.  Are L(V1x...xV4, W) and L(V1, W) x...x L(V4, W) isomorphic vector spaces?

(3) Let R² and R be endowed with their Euclidean metrics. (a) Show that projections p₁(x, y) = x and p₂(x, y) = y are continuous. (b) Show that the direct image of an open set under p₁ or på is open.

(1) Let (X, 7) be a topological space and let A CX. Let TA= {UNA: U ET}. (a) Show that TA is a topology on A. (b) Show that if 7 is Hausdorff, then T|A is Hausdorff.

1 find an o-thogonal bans for the space spanned by bac set {(0,2,1),,0,1), lo,4.0), (4,2,2)} d fi

= Exercise 2. Consider the finite topological space (X, 7) where X and T = {0, {a}, {a,b}, {c,d}, {a, c, d}, {a, b, c, d}, X}. (1) Is this space To? Why or why not? (2) Is this space T₁? Why or why not? (3) What is cl{a}? {a, b, c, d, e}

Could you explain how to show 6.19 in detail?

How should I prove 2.22? Thank you!

Q. Let (X, B(R), µ) be a Borel measure space. If An B = ¢, BnC =¢, µ(ANC) = 0. Determine µ(AUBUC): 1Add file Back Submit ever submit passwordhrough Google Forms. This form was created inside of Lebanese International University Report Abuse Google Forms