Question
please do part d
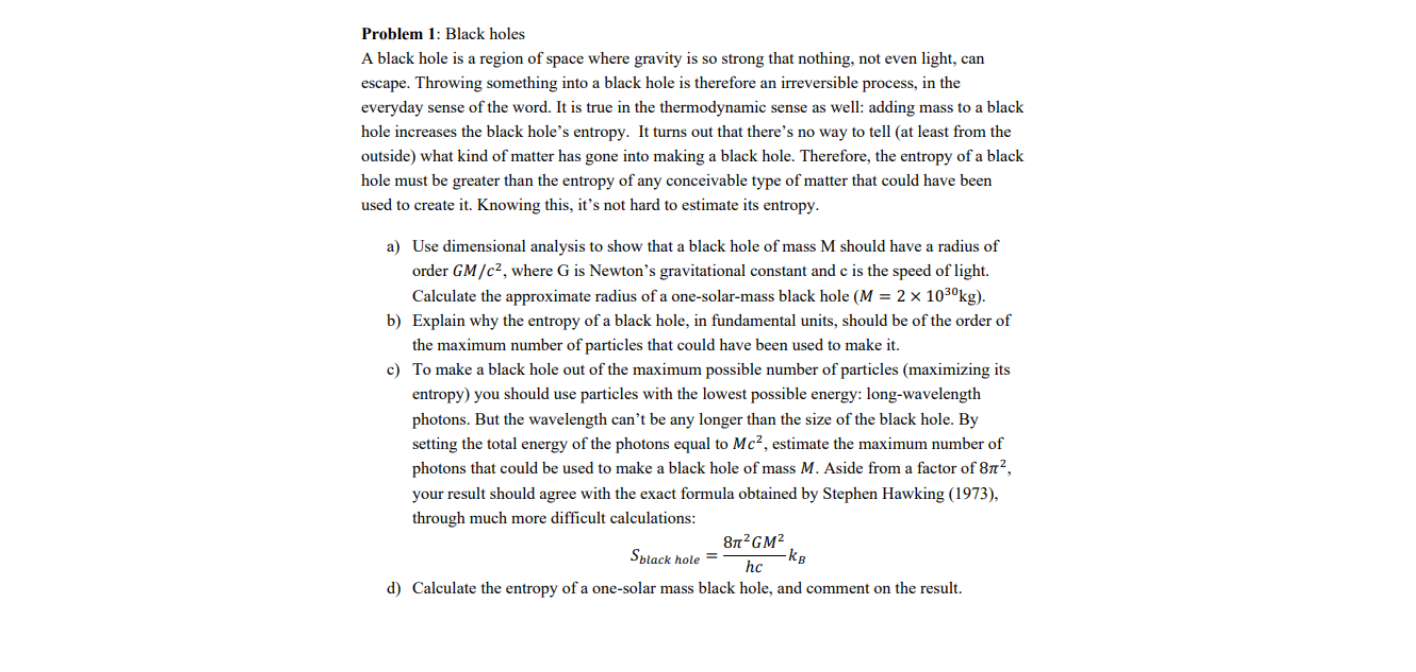
Transcribed Image Text:Problem 1: Black holes
A black hole is a region of space where gravity is so strong that nothing, not even light, can
escape. Throwing something into a black hole is therefore an irreversible process, in the
everyday sense of the word. It is true in the thermodynamic sense as well: adding mass to a black
hole increases the black hole's entropy. It turns out that there's no way to tell (at least from the
outside) what kind of matter has gone into making a black hole. Therefore, the entropy of a black
hole must be greater than the entropy of any conceivable type of matter that could have been
used to create it. Knowing this, it's not hard to estimate its entropy.
a) Use dimensional analysis to show that a black hole of mass M should have a radius of
order GM/c², where G is Newton's gravitational constant and c is the speed of light.
Calculate the approximate radius of a one-solar-mass black hole (M = 2 × 103ºkg).
b) Explain why the entropy of a black hole, in fundamental units, should be of the order of
the maximum number of particles that could have been used to make it.
c) To make a black hole out of the maximum possible number of particles (maximizing its
entropy) you should use particles with the lowest possible energy: long-wavelength
photons. But the wavelength can’t be any longer than the size of the black hole. By
setting the total energy of the photons equal to Mc², estimate the maximum number of
photons that could be used to make a black hole of mass M. Aside from a factor of 8n²,
your result should agree with the exact formula obtained by Stephen Hawking (1973),
through much more difficult calculations:
8n?GM²
-kg
hc
Splack hole =
d) Calculate the entropy of a one-solar mass black hole, and comment on the result.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios