Question
Hello, I need help with PART A, PART B, AND PART C, I don't know how to do these three problems can you help me because it will help to see why I got the answer wrong. Also, can you label which one is Part A, PART B AND PART C
![**Problem 2:**
An early model of the atom, proposed by Rutherford after his discovery of the atomic nucleus, had a positive point charge \(+Ze\) (the nucleus) at the center of a sphere of radius \(R\) with uniformly distributed negative charge \(-Ze\). \(Z\) is the atomic number, the number of protons in the nucleus, and the number of electrons in the negative sphere. Show that the electric field strength inside this atom is
\[ E_{\text{in}} = \frac{Ze}{4\pi\varepsilon_0}\left(\frac{1}{r^2} - \frac{r}{R^3}\right). \]
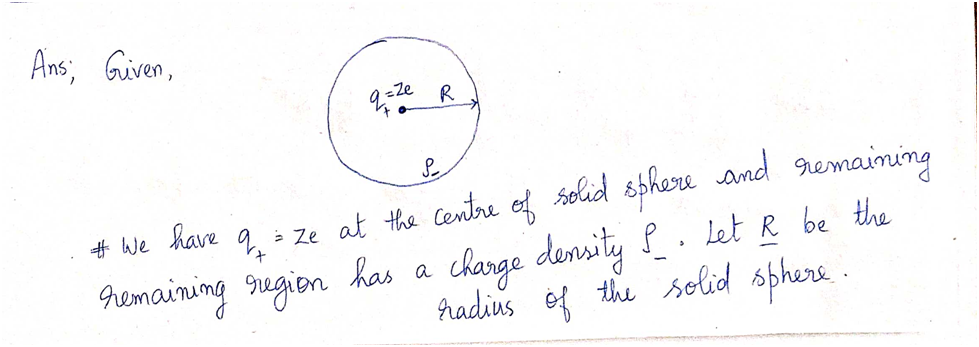
**a)** Fig. 2 illustrates the model of the atom. In the figure, draw the Gaussian surface that you will use to find the field. Draw the vectors that represent the electric field at the Gaussian surface. How do you know the direction of the electric field inside the atom?
---
**Fig. 2:** The scheme for Problem 2
Diagram explanation: The figure shows a circle representing a sphere with a radius \(R\). A small dot in the center represents the positive charge \(Q_+ = Ze\). Around this center, is a dashed line indicating the Gaussian surface. The electric field vectors would point away from the center along the Gaussian surface.
---
**b)** What is the volume charge density \(\rho_-\) of the negative sphere? Use Gauss's law and the symmetry arguments in order to find the expression for the electric field strength inside the atom. What is the electric field strength outside this atom?
**c)** A uranium atom has \(Z = 92\) and \(R = 0.10 \, \text{nm}\). What is the electric field strength at \(r = R/2\)?](https://content.bartleby.com/qna-images/question/135ae25c-4e57-47fe-981c-440929c4b953/7639bbe2-0369-4f58-9327-ad6224a429fd/35og3nh_thumbnail.png)
Transcribed Image Text:**Problem 2:**
An early model of the atom, proposed by Rutherford after his discovery of the atomic nucleus, had a positive point charge \(+Ze\) (the nucleus) at the center of a sphere of radius \(R\) with uniformly distributed negative charge \(-Ze\). \(Z\) is the atomic number, the number of protons in the nucleus, and the number of electrons in the negative sphere. Show that the electric field strength inside this atom is
\[ E_{\text{in}} = \frac{Ze}{4\pi\varepsilon_0}\left(\frac{1}{r^2} - \frac{r}{R^3}\right). \]
**a)** Fig. 2 illustrates the model of the atom. In the figure, draw the Gaussian surface that you will use to find the field. Draw the vectors that represent the electric field at the Gaussian surface. How do you know the direction of the electric field inside the atom?
---
**Fig. 2:** The scheme for Problem 2
Diagram explanation: The figure shows a circle representing a sphere with a radius \(R\). A small dot in the center represents the positive charge \(Q_+ = Ze\). Around this center, is a dashed line indicating the Gaussian surface. The electric field vectors would point away from the center along the Gaussian surface.
---
**b)** What is the volume charge density \(\rho_-\) of the negative sphere? Use Gauss's law and the symmetry arguments in order to find the expression for the electric field strength inside the atom. What is the electric field strength outside this atom?
**c)** A uranium atom has \(Z = 92\) and \(R = 0.10 \, \text{nm}\). What is the electric field strength at \(r = R/2\)?
Expert Solution
arrow_forward
Step 1
Step by stepSolved in 4 steps with 4 images

Knowledge Booster
Similar questions
- I need help calculating the standard deviation for fall time and calculated acceleration (m/s^2) and the standard deviation for that too. I attached a picture of the chart. Please help me.arrow_forwardI need steps to solve this to understand itarrow_forwardOn the map, let the +x-axis point east and the +y-axis north. An airplane flies at 880 km/h northwestward direction (i.e., midway between north and west). Can you help me visualize the components of the first velocity, and when the plane flies due south at the same velocity? I am afraid I can properly visualize or understand in simple terms what is asked. My point can you help to understand the right angle theorem to the angle.arrow_forward
- Using what's given below, answer the following parts for practice: Ansdrew needs to get produce from the store, which is a distance d=2.87 km east of his condo. Andrew rides his bicycle to the store at a constant speed of v1= 6.19 m/s, and rides back to his condoat the slower speed of v2=2.22 m/s. The figure below is not drawn to scale. Part A: How long, in seconds, does it take for Andrew to reach the store? And how long in both seconds and minutes does the whole trip take? Part B: What total distance, in kilometers, was traveled by Andrew during the whole trip? Part C: What is the magnitude, in kilometers, of Andrew's displacement for the entire trip and what was the direction of his displacement for the entire trip?arrow_forwardPlease look at the picture to determine the answers. Question A: An object travels with the velocity function shown on the left. At t = 0, which of the following best describes the motion of the object? 1. moving in the positive direction and speeding up 2. Moving in the positive direction and slowing down 3. moving in the negative direction and speeding up 4. Moving in the negative direction and slowing down 5. Not moving Question B: What is the object's acceleration at t = 2 s? 1. 0 m/s^2 2. 2 m/s^2 3. -2 m/s^2 4. 4 m/s^2 Question C: What is the object's velocity at t = 6 s ? 1. 0 m/s 2. 1 m/s 3. -1 m/s 4. 6 m/s Question D: What is the object's displacement from t = 0 s to t = 6s? 1. 9 m 2. 1 m 3. -1 m 4. It can not be determined from the given information Please answer all of the questionsarrow_forwardA jet plane travels towards a ground-based radar dish. Radar locates the jet plane at a distance D = 11 km from the dish, at an angle θ = 46° above horizontal. 1.) What is the jet plane’s horizontal distance, DH in meters, from the radar dish? 2.)What is the jet plane's vertical distance, DV in meters, above the radar dish? 3.)Write an expression for the distance vector, D, in rectangular form in terms of D and θ, in a coordinate system with the dish at the origin and the unit vectors i and j in the horizontal and vertical directions. (to the right and up)arrow_forward
arrow_back_ios
arrow_forward_ios