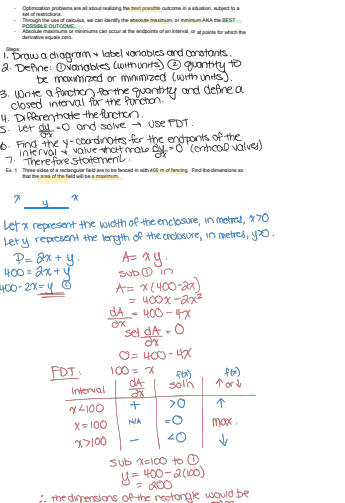
Optimization problem are all about realizing the best possible outcome in a situation, subject to a identify the absolute maximum or minimumAKAST SELE OUTCO derivative equ can can occur at the endpoints of an interval, or at points for which 1. Draw a diagram label variables and constants. 2. Define: Ovanables (with units) quantity to be maximized or minimized (with units). 3. Write a fonction for the quantity and define a closed interval for the function. 4. Differentiate the function. 3. Let dy - and solve Use PDT. 6. Find the y-coordinates for the endpoints of the interval value that make - Contical valves) 7. Therefore statemenil. Ex. 1 Three sides of a rectangular field fenced in with 400 m offencing. Find the dimensions x x Let x represent the width of the enclosure, in metres, x70 Lety represent the length of the enclosure, in metres, yoo. P=2x+y₁ 400=2x+y 400-2x=4 A= xy. Subin A= x(400-2x) = 400x-2x² dA όχι 400-47 set dA-O όχ 0=400-4 FDT 100 = x fox) fox) αA Interval Solh Ford ax 14100 + > x=100 N/A max. 07100 - <0 Sub x=100 to ①. y=400-2(100) = 00 the dimensions of the rectangle would be

A poster consists of a printed area and margins(See image). The area of the entire poster is A = 600 square inches. The goal is to find the dimensions and y that maximizes the printed area. A = 3" The area of the printed part, as a function of x and y is Now, A'(x) = X = y = Use the substitution principle to rewrite the area as a function of x. Hint: You are given the area of the total poster. A = A(x) = To determine optimal values, we need to look at A'(x) = 0. Solving for x and, ultimately for y, yields inches inches 2" X O 2" one ore. te y What is the maximum area of the printed part? A = C 3" square inches

1. A liquid starts at an initial temperature of 175 C and cools down in a room held at a constant temperature of 16 C. It's temperature can be modeled, as a function of time cooling, by the equation y=a(b) +c. which of the following statements is true? (1) a=159 andc=16 (3) a=175 andc=16 (2) a=16 andc=159 (4) a=16 and c=175 14

A standard "money demand" function used by macroeconomists has the form In(m) = Bo + B, In(GDP) + B2R, Where m is the quantity of (real) money, GDP is the value of (real) gross domestic product, and R is the value of the nominal interest rate measured in percent per year. Supposed that B, = 2.15 and B, = -0.06. %3D What is the expected change in m if GDP increases by 3%? The value of m is expected to V by approximately%. (Round your response to the nearest integer) What is the expected change in m if the interest rate increases from 1% to 9%? The value of m is expected to ▼ by approximately %. (Round your response to the nearest integer)