O A = 2 [10] O A = Row 3 expansion: ay аза Суа 1 с 1 2 O 2 J O T O 2₁ +2²₂ 2₂ 2 ( 22 = ( 1 O () Find using 02/²3₁ + ast + O Find elementary get D 00 determinant of Matrix A co factor expansion of Row 3 2 22 1 ට R₂ + R₂ (-1) +R₂ 2 22 -20 02 05₂ ²₁₂ + ax ²0₁3 = Determinant of Matrix. A using Row / column operations first to a triangular Matrix ی 63 |--1-1) C 1 (-2): 1 (2) =/12/ upper triangle det (A)= -1(2)(2) C 9 la

Hi, 

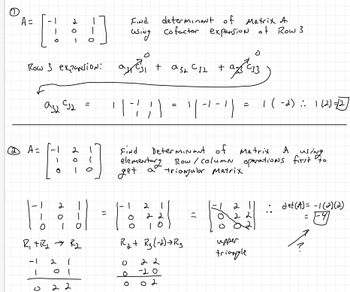
See the attachment.  I'm trying to calculate a determinant of a matrix using two different methods.  First by cofactor expansion, and second by elementary row/column operations producing an upper triangle matrix (solving then by the product of the main diagonal). 

I know the answer is 2, which I got correct using cofactor expansion, but when I try using row operations I get -4 (which is wrong).  Shouldn't I get the same answer regardless of the method I use?  I must not be doing the row operations correctly or my arithmetic is off?

Thank you for the help