
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
question from ablowitz and folkas.
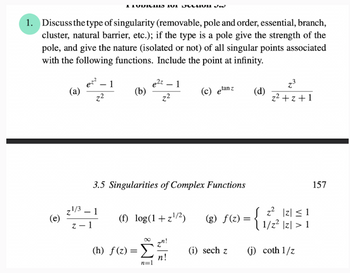
Transcribed Image Text:### Problems for Section 3.5
1. **Discuss the type of singularity** (removable, pole and order, essential, branch, cluster, natural barrier, etc.); if the type is a pole, give the strength of the pole, and give the nature (isolated or not) of all singular points associated with the following functions. Include the point at infinity.
- (a) \(\frac{e^{z^2} - 1}{z^2}\)
- (b) \(\frac{e^{2z} - 1}{z^2}\)
- (c) \(e^{\tan z}\)
- (d) \(\frac{z^3}{z^2 + z + 1}\)
- (e) \(\frac{z^{1/3} - 1}{z - 1}\)
- (f) \(\log(1 + z^{1/2})\)
- (g) \(f(z) = \begin{cases}
z^2 & |z| \leq 1 \\
1/z^2 & |z| > 1
\end{cases}\)
- (h) \(f(z) = \sum_{n=1}^{\infty}\frac{z^n}{n!}\)
- (i) \(\text{sech } z\)
- (j) \(\text{coth }\frac{1}{z}\)
### Notes:
- **Singularity Types**:
- **Removable**: Singularities that can be removed by defining the function appropriately at that point.
- **Pole**: Points where a function goes to infinity. The order of the pole is the highest power in the denominator that causes the infinity.
- **Essential**: Singularities where the function behaves erratically and does not have a pole or removable form.
- **Branch**: Points that introduce a multi-valued nature to functions, like square roots or logarithms.
- **Cluster**: Points where singularities accumulate.
- **Natural Barrier**: Points beyond which the function cannot be analytically continued.
- **Points at Infinity**: Analysis of the behavior of functions as \( z \) approaches infinity is crucial in complex analysis.
Each function listed has its unique behaviors and singular points
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 3 images

Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
need bits after (d)
Solution
by Bartleby Expert
Follow-up Questions
Read through expert solutions to related follow-up questions below.
Follow-up Question
need bits after (d)
Solution
by Bartleby Expert
Knowledge Booster
Similar questions
- 2) Sheila scored 75, 80, 100, 60, and 85 on her last five history tests. She has a final exam that will count as two exams. What score must Shiela obtain on this final exam in order to earn the grade of B? the grading scale for B is 80-89.arrow_forwardSimplify.arrow_forwardThe results of a survey of visitors at the Eiffel Tower showed that 25 spoke English, 15 spoke French, and 8 spoke both English and French. How many spoke English or French?arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

