
Algebra and Trigonometry (6th Edition)
6th Edition
ISBN: 9780134463216
Author: Robert F. Blitzer
Publisher: PEARSON
expand_more
expand_more
format_list_bulleted
Question
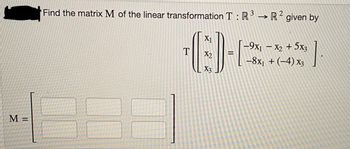
Transcribed Image Text:M =
Find the matrix M of the linear transformation T: R³ → R² given by
3
2
X1
=
(3)-4
X3
T
-9x1 - x₂ + 5x3
-8x₁ + (-4) X3
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 3 images

Knowledge Booster
Similar questions
- M = Find the matrix M of the linear transformation T: R³ → R² given by X1 -C) T X2 X3 12 + (-6) Xx3]. + (-6) x3 -6x1 - x2 -6x₁ + 4x3arrow_forwardCompute the standard matrix for the linear map T : R² → R² which (1) rotates i by 0 = T, (2) stretches the x-component by 3 and (3) reflects the result of (2) about the T - axis.arrow_forwardThe matrices 4₁ = [18] [18], 4₂ = [1] A3 = [8] A4 7 " = - [8], form a basis for the linear space V = R2x2. Write the matrix of the linear transformation T: R²2 R²x2 such that T(A) = 14A + 9AT relative to this basis.arrow_forward
- A linear transformation T : R2 → R2 first reflects pointsabout the y-axis, then rotates points counterclockwise by 45 degrees.(a) Find the standard matrix of T. (b) Find the preimage of (−1, 4).arrow_forward(4) Let B be the matrix 1 1 B = 2 3 4 -1 -3 1 -6 -2 and let TB : R4 → R³ be the linear transformation TB(x) = Bx.arrow_forwardFind the matrix M of the linear transformation T: R³ → R² given by T X1 x2 x 3 7x₁x₂ + (-4) x3 -4x1 + (-8) x₂arrow_forward
- Assume that T is a linear transformation. Find the standard matrix of T. T: R2→R*, T(e,) = (8, 1, 8, 1), and T(e2) = (-6, 9, 0, 0), where e, = (1,0) and e, = (0,1).arrow_forwardDiagonalize the quadratic form by finding an orthogonal matrix Q such that the change of variable x- Qy transforms the given form into one with no cross-product Enter your answer in the form (Q. f(y)) = ([[row 1], [row 2]]. (y)). where each row is a comma- C terms. Give Q and the new quadratic form, f(y). (Assume y separated list and f(y) is in terms of y (Q. (y)) - 6x₁² + 9x₂² Need Help? - 4x₁x2 Read it and Y₂)arrow_forwardA freelance artist is paid $3,520 for a contracted job. The money is invested in a savings account earning 3.05% annual interest compounded monthly. What is the total account balance after 6 months?arrow_forward
- I need helparrow_forwardFind the matrix A of T. A= Consider a linear transformation T from R³ to R² for which = E T = [8]. T = T * (E) - · *(8) - · *(6) - A -H). =arrow_forwardFind the B-matrix for the transformation xAx, where B={b₁,b₂}- -5 - 1 2 = *[*) * * C A = b₁ b₂ 5 The B-matrix of the given transformation isarrow_forward
arrow_back_ios
SEE MORE QUESTIONS
arrow_forward_ios
Recommended textbooks for you
 Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON
Algebra and Trigonometry (6th Edition)AlgebraISBN:9780134463216Author:Robert F. BlitzerPublisher:PEARSON Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning
Contemporary Abstract AlgebraAlgebraISBN:9781305657960Author:Joseph GallianPublisher:Cengage Learning Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning
Linear Algebra: A Modern IntroductionAlgebraISBN:9781285463247Author:David PoolePublisher:Cengage Learning Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON
Algebra And Trigonometry (11th Edition)AlgebraISBN:9780135163078Author:Michael SullivanPublisher:PEARSON Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press
Introduction to Linear Algebra, Fifth EditionAlgebraISBN:9780980232776Author:Gilbert StrangPublisher:Wellesley-Cambridge Press College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education
College Algebra (Collegiate Math)AlgebraISBN:9780077836344Author:Julie Miller, Donna GerkenPublisher:McGraw-Hill Education

Algebra and Trigonometry (6th Edition)
Algebra
ISBN:9780134463216
Author:Robert F. Blitzer
Publisher:PEARSON

Contemporary Abstract Algebra
Algebra
ISBN:9781305657960
Author:Joseph Gallian
Publisher:Cengage Learning

Linear Algebra: A Modern Introduction
Algebra
ISBN:9781285463247
Author:David Poole
Publisher:Cengage Learning

Algebra And Trigonometry (11th Edition)
Algebra
ISBN:9780135163078
Author:Michael Sullivan
Publisher:PEARSON

Introduction to Linear Algebra, Fifth Edition
Algebra
ISBN:9780980232776
Author:Gilbert Strang
Publisher:Wellesley-Cambridge Press

College Algebra (Collegiate Math)
Algebra
ISBN:9780077836344
Author:Julie Miller, Donna Gerken
Publisher:McGraw-Hill Education