Advanced Engineering Mathematics
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
Bartleby Related Questions Icon

Related questions

Q: 6.0. 3) What are the first three terms of the sequence defined by the recursive formulas t₁ = 2 and…
A:
Q: **) Define a sequence a1, a2, a3, ... as follows: a1 = 1, a2 = 3, and a, = an-1+ an-2 for al ategers…
A: The given sequence is 1,3,4,7,11,18,..... such that an=an-1+an-2 We have to prove P(n): an≤(74)n for…
Q: Prove {-23n +5} n=1 to infinity tends to negative infinity. (question is not incomplete, this is the…
A: Given sequence is an=−23n+5. We have to prove that sequence an tends to -∞ that is we have to find…
Q: (M) Find the nth term of the sequence a 4 and lus = 16 10/200/0201/ 20006 b) ₂ = 94 and 16 = 85…
A: Assume the given terms of the sequence is in Arithmetic progression. 3a Given u1=−4 and u5=16. We…
Q: Use induction to prove that 2*3^(n-1) is less than or equal to 2n choose n (picture attached)
A: We have to prove 2⋅3n−1≤2nn by using of principal of mathematical induction, where n≥0 Recall that…
Q: Q4. Use Strong induction to show that Let an be the sequenced defined by 90=0 7 2Step: Case: a =…
A:
Q: Find the 9th term for the sequence of positive 3 digit numbers that are multiples of 5.
A: We have to find the 9th term for the sequence of positive 3 digit numbers that are multiples of 5…
Q: A sequence consists of all 4-digit integers ending in 5, in increasing order. th term. Find the…
A:
Q: Find an increasing subsequence of maximal length and a decreasing subsequence of maximal length in…
A:
Q: A sequence bo, b1, b2, ... is defined by letting bo = 5 and b = 4+bk-1 for each integer k> 1. Show…
A:
Q: Consider the sequence (an) n >0 which begins 3,7,11,15,19,23.. means a0=3.  1.) Find the recursive…
A: 1). Recursive definition is a_(n) =a_(n-1) +4 Because 7=3+4, 11=7+4, 15=11+4
Q: Prove this statement Discrete Math There exists a prime number l so that l^2 − l + 11 is also…
A: We have to prove that, "there exists a prime number I so that I2-I+11 is prime". We know the set of…
Q: Let p and q be distinct primes with p<q and q# 1mod(p). Show that if G|= pq then G Z/pqZ.
A:
Q: Let ao, a1, a2, ... be the sequence defined recursively by an = 7(an-1)² if n ≥ 1 ao = 1, Use the…
A: Principle of mathematical induction states that a statement P(n) is true for all natural numbers n…
Q: Concerning the Fibonacci sequence, use induction to prove that + F2 = F₂Fn+1 F² + F² +F² + -
A:
Q: Show that the sequence delened by +5 mcreasing and ancg"for allkn an is thereas and anz9
A:
Q: Show that 10n <3n for n ≥no. Be sure to find no first.
A:
Q: Suppose that bı, b2, bs, is a sequence defined as bk-1+ bk-2 for each integer ... follows: b1 = 4,…
A:
Q: Use polynomial fitting to find the formula for the nth term of the sequence (an)n>1 which starts,…
A: We find First difference, Second difference ,.... upto we get constant difference. From the step…
Q: The Fibonacci sequence (fa)-1,1,2, 3, 5, 8, 13, 21, is defined recursively by fatt-fa fa-t for all n…
A: Given that the Fibonacci sequence fn=1,1,2,3,5,8,13,21,… is defined recursively as: fn+1=fn+fn-1 for…
Q: How can the sum of k * (n choose k) = n(2 to the power of n-1) be proven akgebraically ?
A: Step 1: We have to prove that, Now by the binomial theorem, Now, differentiating the both sides of…
Question
Liminf of the sequence is an = √2n(√2n +4 | 3 + 2/1/20 n √2n) n is a prime number n is not a prime number
expand button
Transcribed Image Text:Liminf of the sequence is an = √2n(√2n +4 | 3 + 2/1/20 n √2n) n is a prime number n is not a prime number
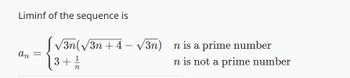
Liminf of the sequence is an √√3n(√3n+4√3n) 3 + n n is a prime number n is not a prime number
expand button
Transcribed Image Text:Liminf of the sequence is an √√3n(√3n+4√3n) 3 + n n is a prime number n is not a prime number
Expert Solution
Check Mark
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
See solutionCheck out a sample Q&A here
Step 1
VIEW
Step 2
VIEW
bartleby

Step by stepSolved in 2 steps with 2 images

See solution
Check out a sample Q&A here
Blurred answer
Knowledge Booster
Background pattern image
Similar questions
Recommended textbooks for you
Text book image
Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated
Text book image
Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education
Text book image
Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY
Text book image
Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,
Text book image
Basic Technical Mathematics
Advanced Math
ISBN:9780134437705
Author:Washington
Publisher:PEARSON
Text book image
Topology
Advanced Math
ISBN:9780134689517
Author:Munkres, James R.
Publisher:Pearson,
SEE MORE TEXTBOOKS