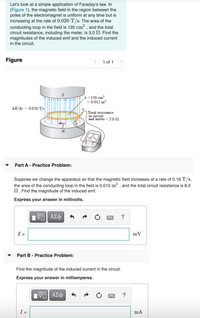
Let's look at a simple application of Faraday's law. In (Figure 1), the magnetic field in the region between the poles of the electromagnet is uniform at any time but is increasing at the rate of 0.020 T/s. The area of the conducting loop in the field is 120 cm² , and the total circuit resistance, including the meter, is 5.0 N. Find the magnitudes of the induced emf and the induced current in the circuit. Figure 1 of 1 A=120 cm =0.012 m AB/A = 0.020 T/2 Total resistance in cireuit and meter= 5.0 - Part A - Practice Problem: Suppose we change the apparatus so that the magnetic field increases at a rate of 0.16 T/s, the area of the conducting loop in the field is 0.015 m² , and the total circuit resistance is 8.3 N. Find the magnitude of the induced emf. Express your answer in millivolts. ? E = mV Part B - Practice Problem: Find the magnitude of the induced current in the circuit, Express your answer in milliamperes. ? I =

Please show all work and explain answer

Find the number of protons and neutron for Po-210. Separate your answer by a comma, indicating the number of protons first before the number of neutrons.

help of these 3 question , I want correct answers please

Could you help with sections B and C?

please also do the calculations