
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
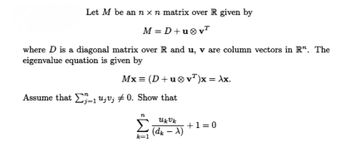
Transcribed Image Text:Let M be an n x n matrix over R given by
M = D+u®v"
where D is a diagonal matrix over R and u, v are column vectors in R". The
eigenvalue equation is given by
Mx = (D+u0 vT)x = Ax.
Assume that u;v; + 0. Show that
Σ
+1= 0
(dr – X)
k=1
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
- Find the first four iterations obtained by the Inverse Power method applied to the matrix A starting with iteration. x (0) = = (-_¹₁), and the linear functional ((*₂)) - =[17¹² 3] = = x₂. Normalize the vector for eacharrow_forwardis an eigenvector of the matrix 2 What is its eigenvalue? Eigenvalue =arrow_forwardShow that the eigenvalues of a real symmetric matrix A = A^T arealways real (use the conjugate transpose).arrow_forward
- Please help me solve this. I got to all three eigens are equal to zero by doing subtraction of 5(first equation)-(third equation) and 3(first equation)-(second equation) and then solced out for my "a" "b" and "c" values. where did I go wrong here?? only HANDWRITTEN answer needed ( NOT TYPED)arrow_forwardFor the matrix A , there is an invertible matrix P such that P^(−1)*AP = D, where D is a diagonal matrix with the eigenvalues of A on the diagonal. Calculate the matrix product P^(-1)*AParrow_forwardSuppose A + u are distinct eigenvalues of A, with corresponding eigenvectors u and v. (Au)™v = Au" v O pu"v V O du7v (Check ALL that apply) uv = are column vectors corresponding to the vectors u, v, then u'v is a Prove your result. Suggestion: If u, matrix whose entry is i · v.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

