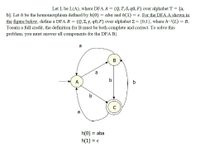
Let L be L(A), where DFA A = (Q,T,8, q0, F) over alphabet T = {a, %3D b}. Let h be the homomorphism defined by h(0) = aba and h(1) = e. For the DFA A shown in the figure below, define a DFAB = (Q,E, y, q0, F) over alphabet E = {0,1}, where h-1(L) = B. Toearn a full credit, the definition for B must be both complete and correct. To solve this problem, you must answer all components for the DFA B.| %3D

Consider the following language: {x ∈ {a, b}∗ |#a(x) ≤ 2#b(x)} (a) Give a CFG for this set.(b) Prove that the given CFG is correct

Computer Science Suppose L ⊆{a, b}∗ is defined as follows: Λ∈L; for every x and y in L, the strings axb, bxa, and x yare in L. Show that L =AEqB , the language of all strings x in {a, b}∗ satisfying n_a (x) =n_b (x).

Mathematical Logic First-order or predicate logic. Show that the sum relation, {(m,n,p)|p=m+n}, is not definable on (N; ∙). Hint: Consider an automorphism of (N; ∙) that interchanges two primes. Where N is the set of natural numbers. Please be as clear as possible. Show and explain all the steps. Thank you very much.

Discrete Structure

Let regular language L1 recognized by DFA (Q1,Σ,δ1,s1,F1) and regular language L2 recognized by DFA (Q2,Σ,δ2,s2,F2). We will construct a product DFA as the quintuple (Q,Σ,δ,s,F) where: •Q=Q1×Q2•For allx∈Σ and (q1,q2)∈Q1×Q2,δ((q1,q2),x) = (δ1(q1,x),δ2(q2,x))•s= (s1,s2) DetermineFso that the product DFA recognizes: (i)L1∪L2(ii)L1∩L2(iii)L1\L2Based on your choice for F, for each case, provide a detailed proof why the product DFA recognizes each of the mentioned languages. Conclude that in-tersection of two regular languages is a regular language and set-theoretic dif-ference of two regular languages is regular.

Write python Code for classifier QMi=1 p(yi|X, α)

Prove that the following languages are not regular using the Pumping lemma and/or closure properties. Note: Do not use other methods. a. L1 = {w ∈ {a, b}∗: na(w) + nb(w) = 3 · nb(w)} (na (w) is used to denote the number of a's in the string w) b. L2 = {anbmck: n, m, k ∈ ℕ, n < m or n < k}

The format P(X) represents as a power set of given "X", (of all X's subsets)   Now, assume there are two sets  A= {q, w, e, r} and B = {w, r, f}. (a) What are the elements of P(A) ∩P(B )?(b) What is the cardinality of P(A ∪B )?(c) What is |P(A) ∪P(B )|?