
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
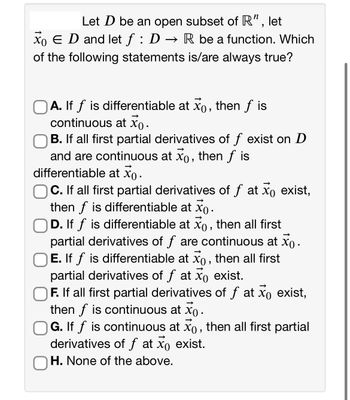
Transcribed Image Text:Let D be an open subset of R", let
XO E D and let f : D → R be a function. Which
of the following statements is/are always true?
OA. If f is differentiable at xo, then f is
continuous at x.
B. all first partial derivatives of ƒ exist on D
and are continuous at x, then ƒ is
differentiable at Xo.
C. If all first partial derivatives of f at xo exist,
then f is differentiable at xo.
D. If f is differentiable at xo, then all first
partial derivatives of f are continuous at xo.
E. If f is differentiable at xo, then all first
partial derivatives of f at xo exist.
F. If all first partial derivatives of f at xo exist,
then f is continuous at xo .
G. If f is continuous at xo, then all first partial
derivatives of f at xo exist.
OH. None of the above.
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
This is a popular solution
Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 47 images

Knowledge Booster
Similar questions
- 4. Show that if f:[0,1]→[0,1] is continuous, then f has at least one fixed point. That is, there exists an xE[0,1] so that f(x) = x.arrow_forward7. Modify B(z) to construct a C function C(z) which satisfies a. C(z) = 0 if S 0.. b. C(z) = 1 if > 1. c. C'(z) > 0 if0< z < 1.arrow_forwardIf a twice differentiable function f has exactly three zeros, at x=1, x=2 and x=3, then which of the following can we necessarily conclude? Select all that apply. O f' has at least two zeros in the interval (1,3) . O f' has exactly one zero in the interval (1,3). O f' is never zero on the interval (1, 3). f" has at least one zero in the interval (1,3). f" is never zero on the interval (1 , 3) .arrow_forward
- Let f : X → Y and g : Y → Z be functions and let B be a subset of Z. Then (g ◦ f)−1(B) = f−1(g−1(B)).arrow_forward4. Let f: [-1, 1] → R be a continuous function. Prove the following statements: (a) If there is c € [-1,1] such that f(c)f(−c) < 0, then there is de R such that f(d) = 0. (b) If ƒ([-1, 1]) = (-1, 1), then f is not continuous.arrow_forward5. In each of the following cases, either write down a function with the specified properties, or explain why no such function exists. (a) An unbounded differentiable function ƒ : [0, 1] → R. (b) A bounded differentiable function f : R → R whose derivative f': R → R is unbounded.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

