
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question

Transcribed Image Text:(b) Apply the Gram-Schmidt procedure to V₁ and v₂ to find an orthogonal basis {u₁, U₂} for W.
U₁
||
U₂
||
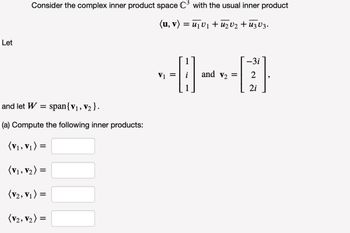
Transcribed Image Text:Let
Consider the complex inner product space C³ with the usual inner product
(u, v) = ₁ V₁ + U₂ V₂ + UzV3.
and let W = span{V₁, V₂}.
(a) Compute the following inner products:
(V₁, V₁ )
(V₁, V₂) =
(V₂, V₁) =
(V2, V₂) =
=
V₁ =
and V₂ =
-3i
2
2i
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 4 steps with 3 images

Knowledge Booster
Similar questions
- T: P2 P2 defined by T(a + bx + cx²) = a + b(x + 8) + b(x + 8)² Find the following images of the function for vectors p₁(x) = a₁ + b₁x + c₁x² and p₂(x) = a₂ + b₂x + c₂x2 in P₂ and the scalar k. (Give all answers in terms of a₁, b₁, C₁, 22, b₂, C₂ and c.) T(P₁) + T(P₂): = T(P1 + P₂) = cT (P1) = T(CP1) = Determine whether T is a linear transformation. 4 Olinear transformation O not a linear transformationarrow_forwardSince it's an if and only if proof, don't you have to prove "if linearly dependent, then scalar multiples" and then "if scalar multiple, then linearly dependent"?arrow_forwardplease helparrow_forward
- Find proj,u and proj, v. Use the Euclidean inner product. u = (1, -2, -1), v = (-1, 2, -1) (a) projyu (b) projvarrow_forward"On R², define the operations of addition and scalar multiplication as follows: (X₁, X₂) + (y₁ Y₂) = (x₂ + y₂ +1, x₂ + y₂ + 1), c(x₁, x₂) = (Cx₂ + C-1, ₂+c-1). Then R² with these operations forms a vector space." Note that here the zero vector is (-1,-1) and the additive inverse of (x1, x2) is (-X₁-2, -x₂-2), not (-x₂-x₂)- a) Show that (-1, -1) acts as the zero vector under these definitions.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

