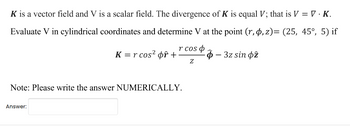
K is a vector field and V is a scalar field. The divergence of K is equal V; that is V = V.K. Evaluate V in cylindrical coordinates and determine V at the point (r, p, z)= (25, 45°, 5) if r cos p K = r cos² pr + Z - 3z sin 02

determine the magnitude and orientation theta of FB so that the resultant force is directed along the positive y axis and has a magnitude of 1500N.

Problem 1.19 Draw a circle in the xy plane. At a few representative points draw the vector v tangent to the circle, pointing in the clockwise direction. By comparing adjacent vectors, determine the sign of avx/ay and avy/ax. According to Eq. 1.41, then, what is the direction of V x v? Explain how this example illustrates the geo- metrical interpretation of the curl.

Please Asap

The force P of magnitude 50 KN is acting at 215° from the x-axis. Find the components of P in u 157° from x, and v negative 69°from x. (Draw the Figure)

ex1

2022, before 4: 06pm. Submit all assignments in my Assignment Box in the Division office. Question 1 oone Find the scalar and vector products of two vectors, (3î – 4j + 5k) and b = a = (2î + j - 3k). Question 2 Use the geometric definition of the cross product and the properties of the cross product to calculate the following: a) (k +j) × (k - i) b) (î + j) × î) × ĵ) c) (4î × (î + ĵ) Question 3 The following external forces are acting on a body: F1 = (10, 2,1)N at point P (4,0,0)cm , %3D F2 = (0,0,5)N at point P2 (1,-3,0)cm and