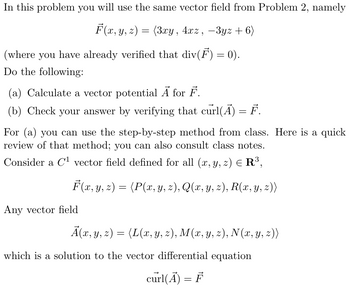
In this problem you will use the same vector field from Problem 2, namely F(x, y, z) = (3xy, 4xz, -3yz+6) (where you have already verified that div(F) = 0). Do the following: (a) Calculate a vector potential Ā for F. (b) Check your answer by verifying that curl(A) = F. For (a) you can use the step-by-step method from class. Here is a quick review of that method; you can also consult class notes. Consider a C¹ vector field defined for all (x, y, z) = R³, F(x, y, z) = (P(x, y, z), Q(x, y, z), R(x, y, z)) Any vector field A(x, y, z) = (L(x, y, z), M(x, y, z), N(x, y, z)) which is a solution to the vector differential equation curl(A) = F is called a vector potential for F. (We know from class or from Briggs calculus that div(curl(A)) 0, so only a vector field with divergence 0 = can have a vector potential; for this problem, you have already calculated that div(F) = 0 in problem 2). Here is a procedure to compute a vector potential: = Starting from A (L, M, N) where L, M, N are unknown, write the formula for curl(Ã), set it equal to ♬ = (P,Q,R), and separate into three component formulas: one for P, one for Q, and one for R. • Assume L = 0, and use assumption that to simplify the component formulas for P, Q and R. Now you know L. Starting from the formula for Q in step (b), partially integrate with respect to x to get a formula for N, having an integration constant f(y, z) that depends on y and z. Assume f(y, z) = 0. Now you know N. Starting from the formula for R in step (b), partially integrate with respect to x to get a formula for M. You should again have an integration constant g(y, z) that again depends on y and z. This time do NOT assume that g(y, z) is equal to 0. Now you partly know M, except you don't yet have a formula for g(y, z). Starting with the formula for P in step (b), -plug in your formulas for N in step (c) -plug in your formula for M in step (d). - Simplify and solve for g(y, z). (There should be no dependence on x in your formula for g(y, z); if there is, you've done something wrong, so go back and check through your work). Now you know M completely. ⚫ Putting it altogether, you've found a formula for a vector potential A = (L, M, N) of the vector field F.

B = ①check that div (B) = 0 ②Find a vector potential for B.

A net is dipped in a river. Determine the flow rate of water across the net if the velocity vector field for the river is given by v=(x-y,z+y+7,z2) and the net is decribed by the equation y=1-x2-z2, y20, and oriented in the positive y- direction. (Use symbolic notation and fractions where needed.)

Suppose that r1(t) and r2(t) are vector-valued functions in 2-space. Explain why solving the equation r1(t)=r2(t) may not produce all the points where the graphs of these functions intersect.  Please Provide Unique Answer. Thank you!

2) Let 7(t) = (t, t², e'). Find an equation for the unit tangent vector T(t).

A particle is moving with velocity V(t) = ( pi cos (pi t), 3t2+ 1) m/s for 0 ≤ t ≤ 10 seconds. Given that the position of the particle at time t = 2s is r(2) = (3, -2), the position vector of the particle at t is?

Suppose f(x, y) = sin (a) ▼ f(x, y) = = 2y X 2y cos 2y 2y 2 cos (2) (2) X - X (b) ▼ƒ(3, π) = 77, 17/0 9' and u is the unit vector in the direction of (1, 1). Then, V (c) fu (3, π) = Du ƒ(3, π) = 0

I am confused on this question. I took the derivative of each section and plugged in 4 and it told me a line cannot be a constant vector. I got L(t)=<10, -1/4, 1>. I am not quit understanding.

Answer both remaining part in 10 minutes in the order to get positive feedback

4. Consider the motion of a particle as described by 1 1 r(t) = = t, Write the acceleration vector of this particle at time t = 1 as a = a+T+ anÑ. You do not need to find T and Ñ, but you can if you find it helpful.