
MATLAB: An Introduction with Applications
6th Edition
ISBN: 9781119256830
Author: Amos Gilat
Publisher: John Wiley & Sons Inc
expand_more
expand_more
format_list_bulleted
Question
Solve for F
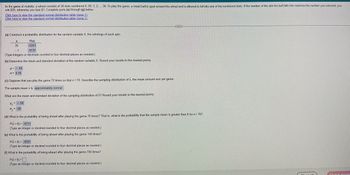
Transcribed Image Text:In the game of roulette, a wheel consists of 38 slots numbered 0, 00, 1, 2,..., 36. To play the game, a metal ball is spun around the wheel and is allowed to fall into one of the numbered slots. If the number of the slot the ball falls into matches the number you selected, you
win $35; otherwise you lose $1. Complete parts (a) through (g) below.
Click here to view the standard normal distribution table (page 1).
Click here to view the standard normal distribution table (page 2).
(a) Construct a probability distribution for the random variable X, the winnings of each spin.
P(x)
0263
X
35
- 1
9737
(Type integers or decimals rounded to four decimal places as needed.)
(b) Determine the mean and standard deviation of the random variable X. Round your results to the nearest penny.
μ= - 05
0 = 5.76
(c) Suppose that you play the game 70 times so that n = 70. Describe the sampling distribution of x, the mean amount won per game.
The sample mean x is approximately normal.
What are the mean and standard deviation of the sampling distribution of x? Round your results to the nearest penny.
E
P = .05
ox
σ- = .69
(d) What is the probability of being ahead after playing the game 70 times? That is, what is the probability that the sample mean is greater than 0 for n = 70?
P(x > 0) = 4711
(Type an integer or decimal rounded to four decimal places as needed.)
(e) What is the probability of being ahead after playing the game 140 times?
P(x > 0) = 4591
(Type an integer or decimal rounded to four decimal places as needed.)
(f) What is the probability of being ahead after playing the game 700 times?
P(x>0)=
(Type an integer or decimal rounded to four decimal places as needed.)
Cloor all
Check answe
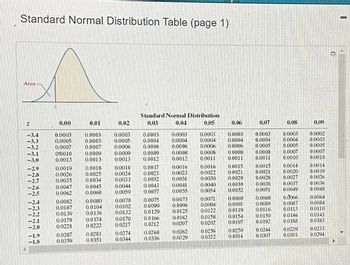
Transcribed Image Text:Standard Normal Distribution Table (page 1)
Area
-34
-3.3
-3.2
-2.1
-1.9
-1.8
0,00
0.0003
0.0005
0.0007
0.0010
0.0013
0.0019
0.0026
0.0035
0.0047
0.0062
0.0082
0.0107
0.0139
0.0179
0.0228
0.0287
0.0359
0.01
0.0003
0.0005
0.0007
0.0009
0.0013
0.0018
0.0025
0.0034
0.0045
0.0060
0.0080
0.0104
0.0136
0.0174
0.0222
0.0281
0.0351
0.02
0.0003
0.0005
0.0006
0.0009
0.0013
0.0018
0.0024
0.0033
0.0044
0.0059
0.0078
0.0102
0.0132
0.0170
0.0217
0.0274
0.0344
Standard Normal Distribution
0.03
0.04
0.05
0.0003
0.0004
0.0006
0.0000
0.0012
0.0017
0.0023
0.0032
0.0043
0.0057
0.0075
0.0099
0.0129
0.0166
0.0212
0.0268
0.0336
0.0003
0.0004
0.0006
0.0008
0.0012
0.0016
0.0023
0.0031
0.0041
0.0055
0.0073
0.0096
0.0125
0.0162
0.0207
0.0262
0.0329
0.0003
0.0004
0.0006
0.0008
0.0011
0.0040
0.0054
0.0071
0.0094
0.0122
0.0158
0.0202
0.06
0.0016
0.0015
0.0022 0.0021
0.0030
0.0029
0.0039
0.0052
0.0256
0.0322
0.0003
0.0004
0.0006
0.0008
0.0011
0.0069
0.0091
0.01 19
0.0154
0.0197
0.0250
0.0314
0.07
0.0003
0.0004
0.0005
0.0008
0.0011
0.0015
0.0021
0.0028
0.0038
0.0051
0.0068
0.0089
0.0116
0.0150
0.0192
0.0244
0.0307
0.08
0.0003
0.0004
0.0005
0.0007
0.0010
0.0014
0.0020
0.0027
0.0037
0.0049
0.0066
0.0087
0.0113
0.0146
0.0188
0.0239
0.0301
0.09
0.0002
0.0003
0.0005
0.0007
0.0010
0.0014
0.0019
0.0026
0.0036
0.0048
0.0064
0.0084
0.0110
0.0143
0.0183
0.0233
0.0294
n
I
Expert Solution
This question has been solved!
Explore an expertly crafted, step-by-step solution for a thorough understanding of key concepts.
Step by stepSolved in 3 steps with 16 images

Knowledge Booster
Similar questions
Recommended textbooks for you
 MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc
MATLAB: An Introduction with ApplicationsStatisticsISBN:9781119256830Author:Amos GilatPublisher:John Wiley & Sons Inc Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning
Probability and Statistics for Engineering and th...StatisticsISBN:9781305251809Author:Jay L. DevorePublisher:Cengage Learning Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning
Statistics for The Behavioral Sciences (MindTap C...StatisticsISBN:9781305504912Author:Frederick J Gravetter, Larry B. WallnauPublisher:Cengage Learning Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON
Elementary Statistics: Picturing the World (7th E...StatisticsISBN:9780134683416Author:Ron Larson, Betsy FarberPublisher:PEARSON The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman
The Basic Practice of StatisticsStatisticsISBN:9781319042578Author:David S. Moore, William I. Notz, Michael A. FlignerPublisher:W. H. Freeman Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman
Introduction to the Practice of StatisticsStatisticsISBN:9781319013387Author:David S. Moore, George P. McCabe, Bruce A. CraigPublisher:W. H. Freeman

MATLAB: An Introduction with Applications
Statistics
ISBN:9781119256830
Author:Amos Gilat
Publisher:John Wiley & Sons Inc

Probability and Statistics for Engineering and th...
Statistics
ISBN:9781305251809
Author:Jay L. Devore
Publisher:Cengage Learning

Statistics for The Behavioral Sciences (MindTap C...
Statistics
ISBN:9781305504912
Author:Frederick J Gravetter, Larry B. Wallnau
Publisher:Cengage Learning

Elementary Statistics: Picturing the World (7th E...
Statistics
ISBN:9780134683416
Author:Ron Larson, Betsy Farber
Publisher:PEARSON

The Basic Practice of Statistics
Statistics
ISBN:9781319042578
Author:David S. Moore, William I. Notz, Michael A. Fligner
Publisher:W. H. Freeman

Introduction to the Practice of Statistics
Statistics
ISBN:9781319013387
Author:David S. Moore, George P. McCabe, Bruce A. Craig
Publisher:W. H. Freeman