
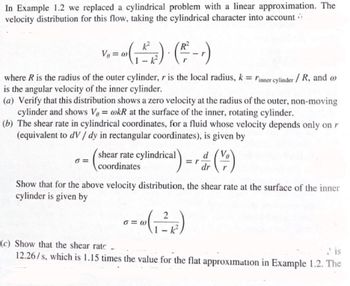
VO is a function of r.
Small r refers to the variable r, as in the radial direction in the cylindrical coordinates. Capital R refers to the radius of the outer cylinder and is a constant. Radius of the inner cylinder is defined as kR, where k (kappa) is also a constant.
In part (a), you are tasked to verify that the profile given in Eqn 1 is correct. You are evaluating the equation at two locations (outer cylinder, inner cylinder).
Recall that angular velocity is w (omega), and w # Ve. VO refers to the local linear velocity in the 0 direction (i.e., tangential velocity).
In part (b), you can ignore the resulting negative sign. You are looking for an expression of the magnitude of the shear rate o (sigma) at the inner cylinder.

Trending nowThis is a popular solution!
Step by stepSolved in 4 steps with 3 images

- 2.8. *The deepest point in the oceans of the world is believed to be in the Marianas Trench, southeast of Japan; there the depth is about 11 000 m. What is the pressure at that point?arrow_forwardShort Answer: When you add salt to water, the boiling point is elevated. Briefly connect this to ideal solutions vs non-ideal solutions and mention how inter-molecular forces are involved. If two components were added together and the resulting mixture approximated an ideal solution, what do you expect to be true about the molecular nature of the two components, do you expect them to be similar or dissimilar, why?arrow_forwardFluid Mechanics easy questionarrow_forward
- 1) Pavithran Ravindran explains to a group of inspiring CBE students that the Reynolds number is a dimensionless group defined for a fluid flowing in a pipe as Re = Dvp/µ where D = pipe diameter, v = average fluid velocity, p = fluid density, and u = fluid viscosity. When the value of the Reynolds number is less than about 2100, the flow is laminar -- the fluid flows in smooth streamlines. For Reynolds numbers above 2100, flow is turbulent -- a great deal of internal agitation takes place. %3D Methyl ethyl ketone (MEK) flows through a 2.067-in. ID pipe at 20°C, at which temperature its density is 0.805 g/cm³ and its viscosity is 0.43 centipoise (1 centipoise = 1.00 x 10-3 kg/m.s). The average fluid velocity is 0.048 ft/s. Determine whether the flow is laminar or turbulent. %3Darrow_forwardConsider the steady, isothermal, irrotational flow of an incompressible frictionless fluid subject only to a body force characterized by a potential O, where F,=- V@ (in units of force/volume). Since the fluid is frictionless, the only surface force is due to pressure, F =-pn. By completing a momentum balance on a material volume of fluid subject to the constraints listed above, derive Bernouilli's law, P + ½pv² + O = constant along a fluid streamline.arrow_forward1. FA Newtonian fluid is flowing between two large parallel plates in the z direction. The top plate is moving in the positive z direction at a constant velocity Vo. The bottom plate is stationary. The velocity profile this flow is given by V(y) = V₁ (a) (b) (77) where 2H is the distance between the two plates. y is the vertical coordinate from the center plane, and is the velocity profile as a function of y. Draw a schematic of the flow system with the appropriate coordinate system. For plates with a total width W in the x-direction and length L in the z-direction, derive an expression for the volumetric flow rate Q through the plates.arrow_forward
 Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education
Introduction to Chemical Engineering Thermodynami...Chemical EngineeringISBN:9781259696527Author:J.M. Smith Termodinamica en ingenieria quimica, Hendrick C Van Ness, Michael Abbott, Mark SwihartPublisher:McGraw-Hill Education Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY
Elementary Principles of Chemical Processes, Bind...Chemical EngineeringISBN:9781118431221Author:Richard M. Felder, Ronald W. Rousseau, Lisa G. BullardPublisher:WILEY Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
Elements of Chemical Reaction Engineering (5th Ed...Chemical EngineeringISBN:9780133887518Author:H. Scott FoglerPublisher:Prentice Hall
 Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning
Industrial Plastics: Theory and ApplicationsChemical EngineeringISBN:9781285061238Author:Lokensgard, ErikPublisher:Delmar Cengage Learning Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The
Unit Operations of Chemical EngineeringChemical EngineeringISBN:9780072848236Author:Warren McCabe, Julian C. Smith, Peter HarriottPublisher:McGraw-Hill Companies, The





