Answered: If f and g are linear transformations…

If f and g are linear transformations are represented by the matrices (0 -4/2 1) and (3 3/-5 -4), what is the image of the point (-1,1) under the composite transformation g o f?

Advanced Engineering Mathematics

10th Edition

ISBN:9780470458365

Author:Erwin Kreyszig

Publisher:Erwin Kreyszig

Chapter2: Second-order Linear Odes

Section: Chapter Questions

Problem 1RQ

See similar textbooks

Related questions

Q: F:R³ → R² defined by ED- 3x1 + x2 - 6x3] -X1 + x2 + 13x3] F

A: For linear transformation 1)Tx+y=Tx+Ty2)Tcx=cTx Given linear transformation is defined as…

Q: 2a + 56+ c -a – 36 – 2c) 3a + 7b +c -4a – 96 - c, 2. T(а + bx + сх*).

A: The solution is given by using rank nullity theorem and null space as follows :

Q: Let T be a linear transformation from R³ into R³. Find T-1 T(x1,x2₁x3)=(2x₁-X3, X₁ + X₂ X3, X2-3x3)…

Q: Find all x in R* that are mapped into the zero vector by the transformation x→A 1-3 1 - 6 1-3 4 -4…

A: (As per the guidelines I am answering only one question at a time)We have to solve for Ax=0where

Q: IfT: R³ R³ is a linear transformation such that --- then T 5 → 0-80-80-4 T

Q: Show that Tis a linear transformation by finding a matrix that implements the mapping. Note that x1,…

A: Let us consider the transformation T: V→W . Consider the standard basis of V is v1,v2,.....vn then…

Q: Write the transformation matrix for a R2→ℝ2 linear transformation that rotates points 150∘ above the…

Q: 3. Each of J, K, L, M and N is a linear transformation from R2 to R?. These functions are given as…

A: Since the question has multiple parts but according to guidelines we will solve the first three sub…

Q: Let S be a linear transformation from R³ to R2 with associated matrix Let T be a linear…

Q: 20x + 20y = 80X20-3 3. Rajah di sebelah menunjukkan susunan gambar di dalam sebuah bingkai gambar.…

A: The distance between the pictures is 2 cm and the distance between the picture and the edge of the…

Q: 3. Prove that ox + 6x -4X12 is irreducible over Q.

Q: If a 2 × 2 matrix A is non-invertible, then the image of the unit square under the linear…

A: False Reason : Here the image of unit square image

Q: Determine whether the following transformations are linear or not. If it is, then find the standard…

A: A transformation L:ℝn→ℝm is said to be linear if it satisfy the condition given below…

Q: -65 -140 30 65 a. If possible, complete the following equations; otherwise, enter DNE. °][³]= [2]…

A: Note that : Since you have posted a question with multiple subparts, we will provide the solution…

Q: Let T be a linear transformation from M, 2 into M, , such that 2,2 Find 4 3 16 -1 4 3

Q: Consider the linear transformation from R² to R² known as “rotation by -90 de- grees." The matrix of…

Q: If T and S are linear transformations such that T: V -> W and S: U -> V, if TS is bijective does…

A: Solution is given below:

Q: Construct a linear fractional transformation that maps 1, -1, 2 to o, i, 0, respectively.

A: Fractional linear transformation

Q: Prove that S + T and cT are linear transformations.

A: Let S and T are linear transformation then we have to prove that S + T and cT are linear…

Q: Write down the matrix of the linear transformation f that maps (1,0) to (3,1) and (0,1) to (-1,1)…

Q: Show that the transformation T defined by T[x1, X2) = (2x, - 3x.2, Xq + 4, 5x,) is not linear. %3D

Q: A transformation is defined by the 2 x 2 matrix —а A a + b а where a and b are scalars. If n is an…

A: Solution: The given matrix is A=-ab-aa+ba where a and b are scalars. We have to prove that for any…

Q: Show that the transformation Y = 2x, + x, + Xg» Y2 = X; + Xg + 2x, ys = x, - 2r, is regular. Write…

Q: Determine whether the function is a linear transformation.

A: The given transformation is T:M3, 3→M3, 3 is defined by TA=001010100A. We have to check whether the…

Q: Let T be a linear transformation from M2,2 into M2,2 such that ([D-[-] ([:D)-[; ²] ([: :)) - [1 ²];…

A: Given :T1000=1-102T0100=0211T0010=1201T0001=3-110

Q: B- Find the transformation matrix of a point [1 01 2] translated by [1 5 0], rotated about x-axis by…

A: From the given information. The given matrix is . The matrix is translated by . The rotation about…

Q: PLS HELP ASAP ON ALL ASKED QUESTIONS PLS

A: Ans 1: (d) Degree of polynomial is 6Ans 2 : (b) Function changes its sign at x =…

Q: Consider the linear transformation from R² to R² known as "projection onto the y-axis." The matrix…

Q: Determine if the following transformation is a linear transformation: 2 x + z X - 3z II

A: Let's check the properties: (1) Tx⇀+z⇀=Tx⇀+Tz⇀: Let x⇀,z⇀ be vectors in R2. Then x⇀=x1x2,z⇀=z1z2,…

Q: Yi 6x1 -1x2 Y2 —17х1 +3x2 I|||

A: Given linear transformation T is y1=6x1-1x2y2=-17x1+3x2 Its inverses is the function T-1 from y to…

Q: ). Find T(1, 3) and 7(1, -5).

Q: Determine whether the following transformation from R3 to R² is linear.

Q: Let R1 and R2 be two 2 × 2 rotation matrices andlet G1 and G2 be two 2 × 2 Givens…

A: Given: R1, R2 are two 2×2 rotation matrices and G1, G2 are two 2×2 givens transformations.To find:…

Q: Let T be a linear transformation from R³ into R³. Find T-1 T(X1₁X₂₁X3) = (X₁ + X3, X₁ −X₂ + X3, X₁ +…

A: Let is a linear transformation from into defined by as follows:.The objective is to determine the…

Q: Find the standard matrix of the composition of linear transformations in R? described by: reflection…

Q: را+6 3-0 * >i

A: Let the mobius transformation be given by f(z) = (az + b)/(cz + d) where a, b, c, d…

Q: Show that T is a linear transformation by finding a matrix that implements the mapping. Note that x,…

Q: Assume that T is a linear transformation. Find the standard matrix of T. T: R² R² first reflects…

A: Under the reflection (0,1) goes to (1,0) and (1,0) goes to (0,1)

Q: Suppose T: P3-M2,2 is a linear transformation whose action on a basis for P3 is as follows: 3]…

Question

If f and g are linear transformations are represented by the matrices (0 -4/2 1) and (3 3/-5 -4), what is the image of the point (-1,1) under the composite transformation g o f?

Expert Solution

Step 1

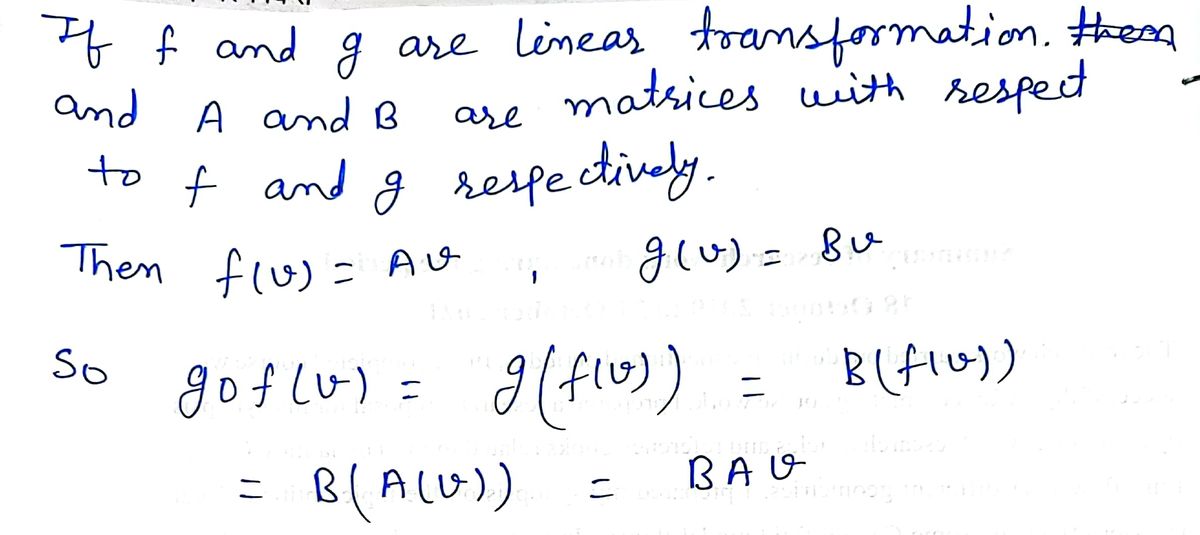
Step by step

Solved in 2 steps with 2 images

SEE SOLUTION Check out a sample Q&A here

Similar questions

Subject:
Let T: R? → P2(R) and U : Rª → M2x2 (R) be linear transformations. A student claims U must be invertible because dim(Rª) = dim(M2x2 (R)). If the student is correct, prove their claim. If the student is not correct, explain why and give an example to illustrate. Clearly state whether or not the student is correct as part of your solution.
Suppose T is a linear transformation, and T((−3, −3, —2, —8, 6, 8)) = (−2, 6, −1,0) The matrix T corresponding to this linear transformation is a by matrix.
2. Write down the matrix of the given linear transformation withrespect to the standard bases of the domain and codomain.
Which of the following is a linear transformation from R2 to R3? a) T(a, b) = (a,0, b²) b) T(а,b) %3D (1, а + b, -b) c) T(a, b) = (a – b, ab, a + b) d) T(a,b) 3D (а, а + b, -а)
Let T1 and T2 be the linear transformations defined by
Answer the following two parts. (a) Find the matrix of the linear transformation, b) Find the matrix of linear transformation that rotates a vector in R2 clockwise by π 3 .
[102] 6. Find the kernel of the linear transformation whose standard matrix is B= 215. [237]
I really need help with this asap please
Determine whether the function is a linear transformation. T: P₂ → P₂, Tao + a₁x + a₂x²) linear transformation O not a linear transformation = (ao + a₁ + a₂) + (a₁ + a₂)x + a2x²
Find the B-matrix for the transformation x-Ax when B=(b₁,b₂, b3}. A= -7 -72 1 17 -4 -72-21 The B-matrix is - 18 - 5, b₁ = -4 -4 ,b₂= -3 4 b3 4 0
Show that the given transformation from R² to R² is linear by showing that it is a matrix transformation. F reflects a vector in the y-axis. Reflecting a vector in the y-axis means negating the x-coordinate. So -| 1819 = [x] F= = and thus F is a matrix transformation with matrix It follows that F is a linear transformation.