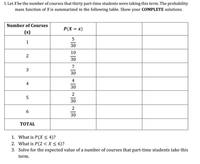
I. Let X be the number of courses that thirty part-time students were taking this term. The probabilitymass function of X is summarized in the following table. Show your COMPLETE solutions.Number of CoursesP(X = x)(x)130102307330443030230ТОTAL1. What is P(X < 4)?2. What is P(2

Answered: Image /qna-images/answer/23f3218f-d720-4498-aad4-89f67c4bf76d.jpg

Answered: I. Let X be the number of courses that…

A First Course in Probability (10th Edition)

10th Edition

ISBN:9780134753119

Author:Sheldon Ross

Publisher:Sheldon Ross

Chapter1: Combinatorial Analysis

Section: Chapter Questions

Problem 1.1P: a. How many different 7-place license plates are possible if the first 2 places are for letters and...

See similar textbooks

Similar questions

Find the wrong answer. The moment generating function Select one: a. is a real-valued function b. is an alternative way to characterize the probability distribution of a random variable c. allows to derive the moments of a random variable d. always exists
Airlines sometimes overbook flights. Suppose that for a plane with 50 seats, 53 passengers have tickets. Define the random variable Y as the number of ticketed passengers who actually show up for the flight. The probability mass function of Y appears in the accompanyingtable: 1. What is the probability that the flight will accommodate all ticketed passengers who showup?2. Find the mean value E(Y ).3. Find the expected value E(−3Y + 1).
Recall the given data. Quality (x) 1 2 Quality (x) 3 Total 1 2 3 Total f(1, 1) = = f(2, 1) = Meal Price (y) f(3, 1) = 1 45 36 9 Each probability f(x, y) will be calculated using the formula below. f(x, y) = number of times x and y occur at the same time total Note that the values along the bottom row and right most column will sum to 1. We are given that a total of 300 restaurants were evaluated. There are 45 instances for a quality rating of x = 1 and a meal price rating of y = 1. Use the given data to complete the table of probabilities. 2 1 42 45 300 3 Total 3 90 57 66 159 12 30 90 111 99 300 51 Meal Price (y) f(1, 2) = f(2, 1) = f(3, 2) = 2 f(1, 3) = f(2, 3) = f(3, 1) = 3 Total 1.00
10 A nature reserve has one bird of an uncommon species which frequents the large expanse of reed beds there. It is found that if a birdwatcher arrives at the path adjoining the reed beds, the probability that he or she is still waiting there to see the bird a time y later, where y is measured in minutes, is ly + 3 y ≥ 0. Find the probability that a birdwatcher spends between 2 and 4 minutes waiting to see the bird, and the mean and variance of the time that he or she waits there.
Of all awarded prizes, 2% are worth $1000, 10% are worth $100, and 88% are worth $10. Let X be the the winnings for a single ticket. Find 1. E(X) (the expected winnings) 2. V(X) 3. E(10X + 2) 4. V(10X + 2)
statistic discrete rondom variables
T3.1 Thank you.
gn X Three couples and two single individuals have been invited to an investment seminar and have agreed to attend. Suppose the probability that any particular couple or individual arrives late is 0.41 (a couple will travel together in the same vehicle, so either both people will be on time or else both will arrive late). Assume that different couples and individuals are on time or late independently of one another. Let X = the number of people who arrive late for the seminar. (a) Determine the probability mass function of X. [Hint: label the three couples #1, #2, and #3 and the two individuals #4 and #5.] (Round your answers to four decimal places.) P(X = X) X 0 1 2 3 4 5 6 7 8 (b) Obtain the cumulative distribution function of X. (Round your answers to four decimal places.) F(x) X 0 1 2 3 4 5 6 7 Use the cumulative distribution function of X to calculate P(2 ≤ x ≤ 5). (Round your answer to four decimal places.) P(2 ≤ x ≤ 5) = Need Help? Read It Watch It 4
The annual rainfall in a certain country varies from 0 to 9 inches and is a random variable with probability density function defined by f(x): - 9.5 x 45 Find the following probabilities for the annual rainfall in a randomly selected year. Round your answers to 4 decimal places as needed. What is the probability that the annual rainfall is greater than 3 inches per year? What is the probability that the annual rainfall is less than 2 inches per year? What is the probability that the annual rainfall is between 2 and 4 inches per year?
A commuter must pass through five traffic lights on her way to work and will have to stop at each one that is red. She estimates the probability model for the number of red lights she hits, as shown below: X= # of red | 0 | 1 | 2 | 3 | 4 | 5 0.05 | 0.25 | 0.35 | 0.15 | 0.15 | 0.05 P(X=x) a. How many red lights should she expect to hit each day? b. What is the standard deviation?
The cost of all maintenance for a car during its first year is approximately exponentially distributed with a mean of $204. a. The lambda of this distribution is b. The probability that the cost is larger than $238 is P(x > $238) = c. The probability that the cost is less than $76 is P(x s $76) = d. The probability that the cost is between $185 and $244 is P($185 s x < $244) = e. The 61st percentile is cost of maintenance s
what is the predicted blood pressure for a man aging 25 years?

SEE MORE QUESTIONS

Recommended textbooks for you

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability (10th Edition)

Probability

ISBN:

9780134753119

Author:

Sheldon Ross

Publisher:

PEARSON

A First Course in Probability

Probability

ISBN:

9780321794772

Author:

Sheldon Ross

Publisher:

PEARSON

SEE MORE TEXTBOOKS