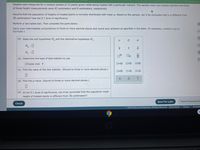
Heights were measured for a random sample of 12 plants grown while being treated with a particular nutrient. The sample mean and sample standard deviation of those height measurements were 42 centimeters and 8 centimeters, respectively. Assume that the population of heights of treated plants is normally distributed with mean u. Based on the sample, can it be concluded that u is different from 36 centimeters? Use the 0.1 level of significance. Perform a two-tailed test. Then complete the parts below. Carry your intermediate computations to three or more decimal places and round your answers as specified in the table. (If necessary, consult a list of formulas.) (a) State the null hypothesis H and the alternative hypothesis H,. H, :0 H, :0 (b) Determine the type of test statistic to use. (Choose one) OSO (c) Find the value of the test statistic. (Round to three or more decimal places.) O

Equations and inequalities describe the relationship between two mathematical expressions.

A linear function can just be a constant, or it can be the constant multiplied with the variable like x or y. If the variables are of the form, x2, x1/2 or y2 it is not linear. The exponent over the variables should always be 1.