
Advanced Engineering Mathematics
10th Edition
ISBN: 9780470458365
Author: Erwin Kreyszig
Publisher: Wiley, John & Sons, Incorporated
expand_more
expand_more
format_list_bulleted
Question
Given a basis T =
{[ 1 0
0 1 ]
,
[1 1
0 −1]
,
[0 0
1 1 ]
,
[0 0
−1 1 ]}
for M2×2,
(a) find [~v]T for ~v =
[1 −1
7 4 ]
(b) find ~u such that [~u]T =
[−2
−1
3
0]
Expert Solution
arrow_forward
Step 1
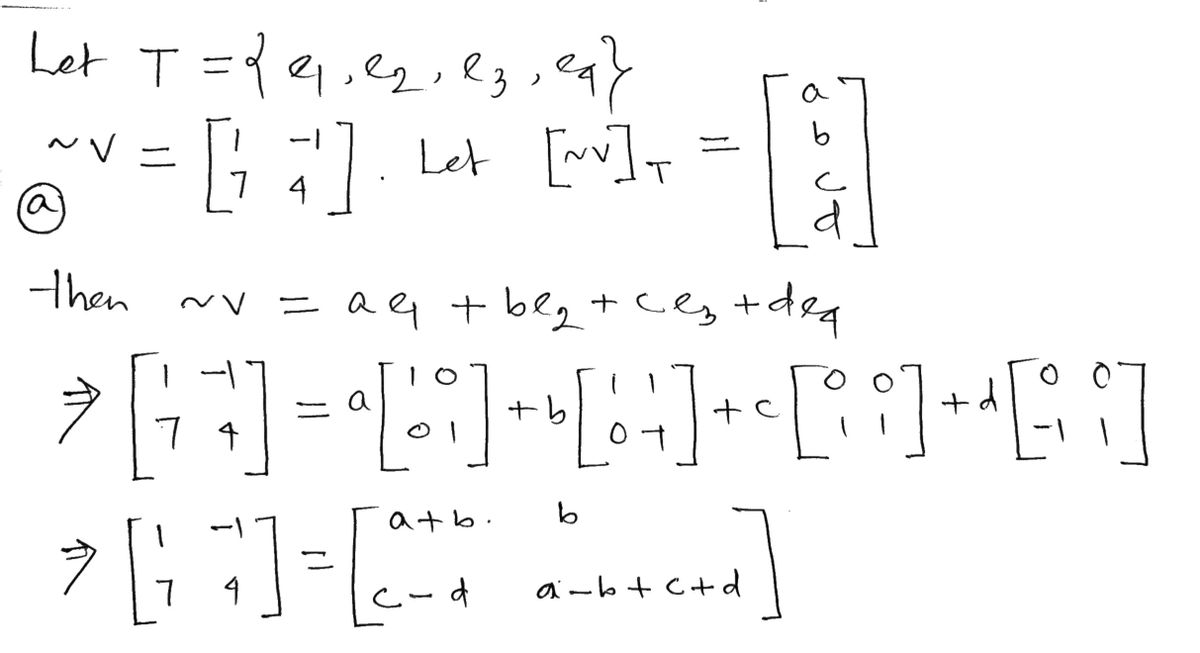
Step by stepSolved in 2 steps with 2 images

Knowledge Booster
Similar questions
- Compute the quadratic form x' Ax for A = X1 5 444 X2 -9 x3 a. x = a. x¹ Ax= b. x' Ax= C. X' Ax= 1/√√3 b. x= 7 C. X = - 1/√3 1/√√3 (Simplify your answer.) 360 643 035 (Simplify your answer.) and each of the following vectors.arrow_forward-13/27] -4/27 -4/9 5/9 -20/27] -2/27 Let v₁ = √2 = and √3 = Find a vector in IR4 such that -20/27 ' ' -2/9 17/27 4/9 -2/3 2/9 {1, 2, 3, 4} is an orthonormal set. Answer:arrow_forwardLet the vector C represents the coefficients of a polynomial pt=c1+c2t+c3t2+c4t3. Express the conditions p0=1, p'0=2, p1=1, p'1=0 in the form AC=b.arrow_forward
arrow_back_ios
arrow_forward_ios
Recommended textbooks for you
 Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated
Advanced Engineering MathematicsAdvanced MathISBN:9780470458365Author:Erwin KreyszigPublisher:Wiley, John & Sons, Incorporated Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education
Numerical Methods for EngineersAdvanced MathISBN:9780073397924Author:Steven C. Chapra Dr., Raymond P. CanalePublisher:McGraw-Hill Education Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY
Introductory Mathematics for Engineering Applicat...Advanced MathISBN:9781118141809Author:Nathan KlingbeilPublisher:WILEY Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,
Mathematics For Machine TechnologyAdvanced MathISBN:9781337798310Author:Peterson, John.Publisher:Cengage Learning,


Advanced Engineering Mathematics
Advanced Math
ISBN:9780470458365
Author:Erwin Kreyszig
Publisher:Wiley, John & Sons, Incorporated

Numerical Methods for Engineers
Advanced Math
ISBN:9780073397924
Author:Steven C. Chapra Dr., Raymond P. Canale
Publisher:McGraw-Hill Education

Introductory Mathematics for Engineering Applicat...
Advanced Math
ISBN:9781118141809
Author:Nathan Klingbeil
Publisher:WILEY

Mathematics For Machine Technology
Advanced Math
ISBN:9781337798310
Author:Peterson, John.
Publisher:Cengage Learning,

